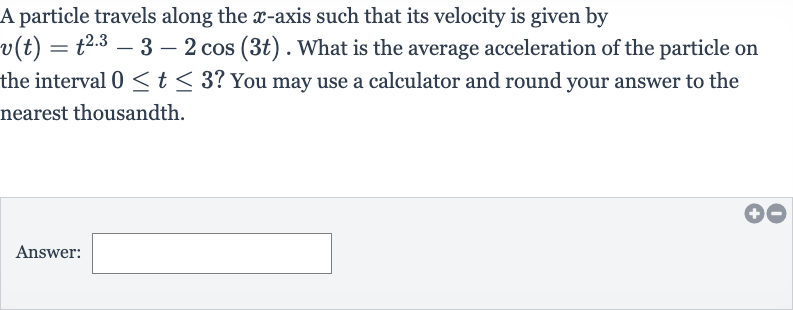Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

A particle travels along the
x-axis such that its velocity is given by
v(t)=t^(2.3)-3-2cos(3t). What is the average acceleration of the particle on the interval
0 <= t <= 3 ? You may use a calculator and round your answer to the nearest thousandth.
Answer:

A particle travels along the $x$ -axis such that its velocity is given by $v(t)=t^{2.3}-3-2 \cos (3 t)$ . What is the average acceleration of the particle on the interval $0 \leq t \leq 3$ ? You may use a calculator and round your answer to the nearest thousandth. $\newline$ Answer:

View step-by-step help

View step-by-step help

Relate position, velocity, speed, and acceleration using derivatives

Full solution

Q. A particle travels along the

x

-axis such that its velocity is given by

v(t)=t^{2.3}-3-2 \cos (3 t)

. What is the average acceleration of the particle on the interval

0 \leq t \leq 3

? You may use a calculator and round your answer to the nearest thousandth.

\newline

Answer:

Calculate Initial Velocity: To find the average acceleration over the interval from $t = 0$ to $t = 3$ , we need to calculate the change in velocity over the change in time. The formula for average acceleration ( $a_{\text{avg}}$ ) is: $\newline$ $a_{\text{avg}} = \frac{v(\text{final}) - v(\text{initial})}{t(\text{final}) - t(\text{initial})}$ $\newline$ First, we need to find the initial and final velocities using the given velocity function $v(t) = t^{2.3} - 3 - 2\cos(3t)$ .
Calculate Final Velocity: Let's calculate the initial velocity $v(0)$ :
$v(0) = 0^{2.3} - 3 - 2\cos(3\cdot 0)$
$v(0) = 0 - 3 - 2\cos(0)$
$v(0) = -3 - 2(1)$
$v(0) = -3 - 2$
$v(0) = -5$ cm/s
Recalculate Cosine Function: Now, let's calculate the final velocity $v(3)$ :
$v(3) = 3^{2.3} - 3 - 2\cos(3 \times 3)$
$v(3) = 3^{2.3} - 3 - 2\cos(9)$
Use a calculator to find the value of $3^{2.3}$ and $\cos(9)$ .
Recalculate Cosine Function: Now, let's calculate the final velocity $v(3)$ :
$v(3) = 3^{2.3} - 3 - 2\cos(3 \times 3)$
$v(3) = 3^{2.3} - 3 - 2\cos(9)$
Use a calculator to find the value of $3^{2.3}$ and $\cos(9)$ .Using a calculator, we find:
$3^{2.3} \approx 20.0855$
$\cos(9)$ is not a standard value, and it seems we have made a mistake. The argument of the cosine function should be in radians, not degrees. Since the problem does not specify, we assume the standard mathematical practice of using radians. We need to recalculate $\cos(3 \times 3)$ correctly.

More problems from Relate position, velocity, speed, and acceleration using derivatives

Question

A particle travels along the

x

-axis such that its velocity is given by

v(t)=t^{0.7} \sin (2 t+3)

. What is the acceleration of the particle at time

t=4

? You may use a calculator and round your answer to the nearest thousandth.

\newline

Posted 9 months ago

Question

A particle travels along the

x

-axis such that its velocity is given by

v(t)=t^{1.1} \cos \left(t^{2}-5\right)

. If the position of the particle is

x=-2

t=2.5

, what is the position of the particle when

t=1

? You may use a calculator and round your answer to the nearest thousandth.

\newline

Posted 9 months ago

Question

A particle travels along the

x

-axis such that its velocity is given by

v(t)=t^{0.9} \sin (t-1)

. If the position of the particle is

x=-3

t=3

, what is the position of the particle when

t=5

? You may use a calculator and round your answer to the nearest thousandth.

\newline

Posted 9 months ago

Question

A particle travels along the

x

-axis such that its velocity is given by

v(t)=t^{2.1} \sin (2 t)

. Find all times when the speed of the particle is equal to

2

on the interval

0 \leq t \leq 4

. You may use a calculator and round your answer to the nearest thousandth.

\newline

t=

Posted 9 months ago

Question

A particle travels along the

x

-axis such that its velocity is given by

v(t)=\left(t^{2.3}+5\right) \sin (2 t)

. What is the distance traveled by the particle over the interval

0 \leq t \leq 5

? You may use a calculator and round your answer to the nearest thousandth.

\newline

Posted 9 months ago

Question

A particle travels along the

x

-axis such that its velocity is given by

v(t)=t^{1.9} \cos (3 t-1)

. What is the distance traveled by the particle over the interval

0 \leq t \leq 3

? You may use a calculator and round your answer to the nearest thousandth.

\newline

Posted 9 months ago

Question

A particle travels along the

x

-axis such that its velocity is given by

v(t)=\left(t^{1.6}+3\right) \cos (3 t)

. What is the average velocity of the particle on the interval

0 \leq t \leq 6

? You may use a calculator and round your answer to the nearest thousandth.

\newline

Posted 9 months ago

Question

A particle travels along the

x

-axis such that its acceleration is given by

a(t)=\left(t^{0.5}+3\right) \cos (3 t)

. If the velocity of the particle is

v=-2

t=1

, what is the velocity of the particle when

t=1

? You may use a calculator and round your answer to the nearest thousandth.

\newline

Posted 9 months ago

Question

A particle travels along the

x

-axis such that its velocity is given by

v(t)=t^{2.1} \sin (2 t+5)

. Find all times when the speed of the particle is equal to

1

on the interval

0 \leq t \leq 3

. You may use a calculator and round your answer to the nearest thousandth.

\newline

t=

Posted 9 months ago

Question

A particle travels along the

x

-axis such that its velocity is given by

v(t)=\left(t^{2.1}+5\right) \cos (2 t)

. What is the distance traveled by the particle over the interval

0 \leq t \leq 5

? You may use a calculator and round your answer to the nearest thousandth.

\newline

Posted 9 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant