AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
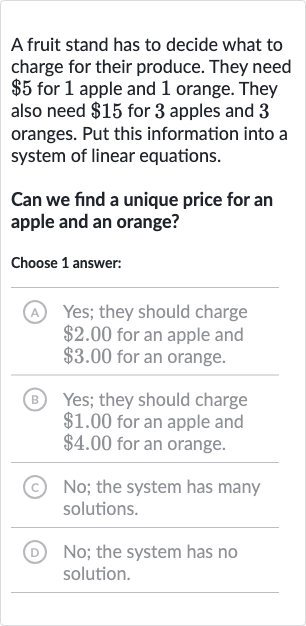
A fruit stand has to decide what to charge for their produce. They need for apple and orange. They also need for apples and oranges. Put this information into a system of linear equations.Can we find a unique price for an apple and an orange?Choose answer:(A) Yes; they should charge for an apple and for an orange.(B) Yes; they should charge for an apple and for an orange.(C) No; the system has many solutions.(D) No; the system has no solution.
Full solution
Q. A fruit stand has to decide what to charge for their produce. They need for apple and orange. They also need for apples and oranges. Put this information into a system of linear equations.Can we find a unique price for an apple and an orange?Choose answer:(A) Yes; they should charge for an apple and for an orange.(B) Yes; they should charge for an apple and for an orange.(C) No; the system has many solutions.(D) No; the system has no solution.
- Translate Equations: Let's denote the price of an apple as and the price of an orange as . The information given can be translated into two equations based on the cost of the fruits: apple + orange = apples + oranges = In terms of and , these equations are: ...() ...()
- Simplify Equation (): Now, let's simplify equation () by dividing each term by to see if it provides any new information:This is the same as equation (), which means that the two equations are actually the same line when graphed. Therefore, they do not provide unique solutions for and .
- Infinite Solutions: Since both equations are the same, there are infinitely many solutions that satisfy both equations. This means that we cannot determine a unique price for an apple and an orange based on the given information alone. The system of equations is dependent and consistent with many solutions.
More problems from Experimental probability
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
