Full solution
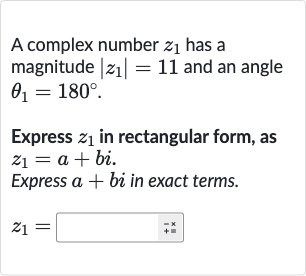
Q. A complex number has a magnitude and an angle .Express in rectangular form, as .Express in exact terms.
- Conversion formulas: A complex number in polar form can be expressed in rectangular form using the conversion formulas and , where is the magnitude and is the angle in radians.
- Convert angle to radians: First, we need to convert the angle from degrees to radians. The angle given is degrees, which is equivalent to radians since degrees radians degrees) radians.
- Calculate rectangular form: Now we can use the magnitude and the angle to find the rectangular form. We calculate and .
- Calculate : Calculating gives us , since .
- Calculate : Calculating gives us , since .
- Final rectangular form: Therefore, the complex number in rectangular form is , which simplifies to .
More problems from Write equations of cosine functions using properties
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help