Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

a and
b are the intercepts made by
a line on the co-ordinate axes. If
3a=b and the line passes through
(1,3), then the equation of the line is

$a$ and $b$ are the intercepts made by a line on the co-ordinate axes. If $3a=b$ and the line passes through $(1,3)$ , then the equation of the line is $\newline$ A) $x+3y =10$ $\newline$ B) $3x+y =6$ $\newline$ C) $x-3y+8 =0$ $\newline$ D) $3x-2+3 =0$

View step-by-step help

View step-by-step help

Transformations of linear functions

Full solution

Q.

a

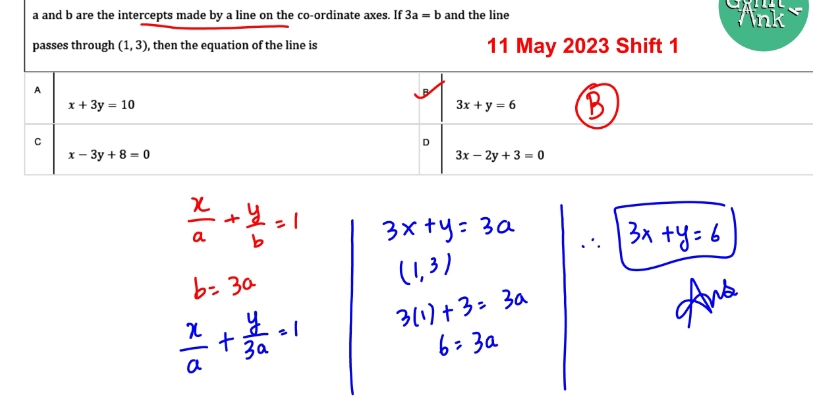
and

b

are the intercepts made by a line on the co-ordinate axes. If

3a=b

and the line passes through

(1,3)

, then the equation of the line is

\newline

A)

x+3y =10

\newline

B)

3x+y =6

\newline

C)

x-3y+8 =0

\newline

D)

3x-2+3 =0

Identify Relationship: Identify the relationship between the intercepts $a$ and $b$ . Given that $3a = b$ , we can express $b$ in terms of $a$ . Calculation: $b = 3a$
Determine Equation: Determine the equation of the line using intercept form. $\newline$ The intercept form of a line's equation is $\frac{x}{a} + \frac{y}{b} = 1$ . $\newline$ Since we know $b = 3a$ , we can substitute $b$ in the equation. $\newline$ Calculation: $\frac{x}{a} + \frac{y}{(3a)} = 1$
Use Given Point: Use the given point $(1,3)$ to find the value of $a$ . $\newline$ Substitute $x = 1$ and $y = 3$ into the equation $\frac{x}{a} + \frac{y}{3a} = 1$ . $\newline$ Calculation: $\frac{1}{a} + \frac{3}{3a} = 1$
Simplify Equation: Simplify the equation to solve for $a$ . Combine the terms on the left side of the equation. Calculation: $\frac{1}{a} + \frac{1}{a} = 1$ Calculation: $\frac{2}{a} = 1$
Solve for a: Solve for a. Multiply both sides by $a$ to isolate $a$ . Calculation: $2 = a$
Find Value of b: Find the value of b using the relationship $b = 3a$ . $\newline$ Substitute $a = 2$ into $b = 3a$ . $\newline$ Calculation: $b = 3 \times 2$ $\newline$ Calculation: $b = 6$
Write Final Equation: Write the final equation of the line using the values of $a$ and $b$ . Substitute $a = 2$ and $b = 6$ into the intercept form equation $\frac{x}{a} + \frac{y}{b} = 1$ . Calculation: $\frac{x}{2} + \frac{y}{6} = 1$
Multiply for Standard Form: Multiply through by the least common multiple of the denominators to get the standard form of the equation. $\newline$ Calculation: $3(\frac{x}{2}) + (\frac{y}{6}) = 3$ $\newline$ Calculation: $\frac{3x}{2} + \frac{y}{6} = 3$ $\newline$ Calculation: $6(\frac{3x}{2} + \frac{y}{6}) = 6(3)$ $\newline$ Calculation: $9x + y = 18$

More problems from Transformations of linear functions

Question

This equation shows how the time required to screen-print a batch of shirts is related to the number of shirts in the batch.

\newline

t = s + 13

\newline

s

represents the number of shirts in the batch, and the variable

t

represents the time required to screen-print the shirts. How long does it take to screen-print a batch of

4

\newline

_________minutes

Posted 2 months ago

Question

Krysta wants to take piano lessons. She asked a teacher how many new pieces she could expect to learn each month. The teacher gave an estimate of

3

. Write an equation that shows how the total number of pieces Krysta would learn,

y

, depends on the number of months of piano lessons,

x

\newline

y

Posted 2 months ago

Question

Tom bought a boat for

\$3,000

and incurs a daily storage fee of

\$15

. Write a linear function to represent the total amount Tom will owe,

p(x)

x

days to pick it up.

\newline

Posted 3 months ago

Question

180

-page book cover to cover in a single session, at a constant rate. It took him

6

\newline

\newline

y

represent the number of pages left to read after

\newline

t

\newline

Which of the following information about the graph of the relationship is given?

\newline

1

\newline

\newline

x

\newline

\newline

y

\newline

(C) Slope and a point that is not an intercept

\newline

\newline

x

\newline

y

\newline

\newline

y

-intercept and a point that is not an intercept

\newline

(F) Two points that are not intercepts

Posted 4 months ago

Question

A charity organization had to sell

18

tickets to their fundraiser just to cover necessary production costs. They sold each ticket for

\$45

y

represent the net profit (in dollars) when they have sold

x

tickets. Which of the following information about the graph of the relationship is given?

\newline

1

\newline

x

\newline

y

\newline

(C) Slope and a point that is not an intercept

\newline

x

y

\newline

y

-intercept and a point that is not an intercept

\newline

(F) Two points that are not intercepts

Posted 2 months ago

Question

Hiro painted his room. After

3

hours of painting at a rate of

8

square meters per hour, he had

28

square meters left to paint.

\newline

\newline

y

represent the area (in square meters) left to paint after

\newline

x

\newline

Which of the following information about the graph of the relationship is given?

\newline

1

\newline

\newline

x

\newline

\newline

y

\newline

(C) Slope and a point that is not an intercept

\newline

\newline

x

\newline

y

\newline

\newline

y

-intercept and a point that is not an intercept

\newline

(F) Two points that are not intercepts

Posted 2 months ago

Question

Naoya read a book cover to cover in a single session, at a rate of

55

pages per hour. After

4

350

pages left to read.

\newline

y

represent the number of pages left to read after

x

\newline

Complete the equation for the relationship between the number of pages left and number of hours.

\newline

y=

Posted 1 month ago

Question

A teacher purchased

300

colored pencils for an upcoming project. The pencils she ordered come in packs of

20

30

14

packs in all. Write a system of equations to find the number of

20

(x)

30

(y)

the art teacher ordered.

Posted 2 months ago

Question

C=0.25(L-1200)

\newline

C

, in dollars, to the holder of a liability insurance account given a total liability of

L

dollars is given by the equation as long as

C

is positive. By how many dollars does the liability increase when the cost to the holder increases by

1

Posted 2 months ago

Question

P=\frac{2}{5} m+1.5

\newline

P

, in dollars, of a train ticket used to travel a distance of

m

miles is given by the equation. By how many miles does the traveling distance increase if the ticket price increases by

1

Posted 4 months ago