Full solution
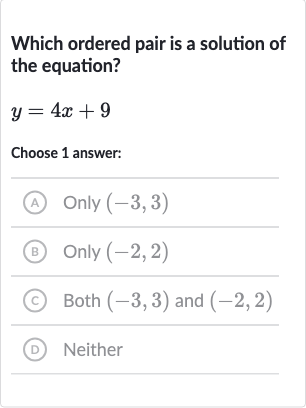
Q. Which ordered pair is a solution of the equation?Choose answer:(A) Only (B) Only (C) Both and (D) Neither
- Problem Understanding: Understand the problem.We need to determine which ordered pair(s) satisfy the equation . To do this, we will substitute the -value of each ordered pair into the equation and check if the resulting -value matches the one given in the pair.
- Testing Ordered Pair : Test the first ordered pair .Substitute into the equation .Calculate : .Check if this y \-value matches the y \-value in the ordered pair .Since the calculated y \-value is and not , the ordered pair is not a solution to the equation.
- Testing Ordered Pair : Test the second ordered pair .Substitute into the equation .Calculate : .Check if this y \-value matches the y \-value in the ordered pair .Since the calculated y \-value is and not , the ordered pair is not a solution to the equation.
More problems from Does (x, y) satisfy the linear equation?
QuestionGet tutor help
QuestionGet tutor help