Full solution
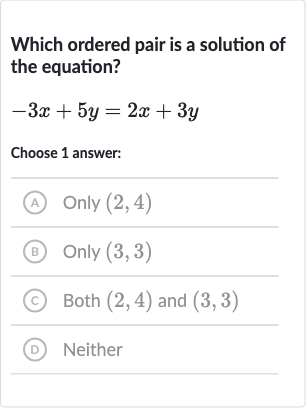
Q. Which ordered pair is a solution of the equation?Choose answer:(A) Only (B) Only (C) Both and (D) Neither
- Combine like terms: Combine like terms by moving the terms to one side and the terms to the other side.
- Simplify the equation: Simplify the equation by combining like terms.
- Test ordered pair : Now we will test each ordered pair to see if it satisfies the equation (-5\)x + y = .First, let's test the ordered pair .Substitute and into the equation.Since (-2\)\ does not equal (0\), the ordered pair is not a solution.
- Test ordered pair : Next, let's test the ordered pair .Substitute and into the equation.Since does not equal , the ordered pair is not a solution.
- No solution found: Since neither nor satisfy the equation , the correct answer is Neither.
More problems from Does (x, y) satisfy the linear equation?
QuestionGet tutor help
QuestionGet tutor help