AI tutor
Full solution
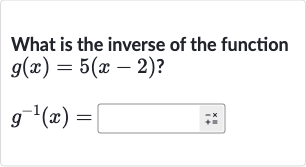
Q. What is the inverse of the function
- Concept of Inverse Function: Understand the concept of an inverse function.The inverse function will undo the operation done by . To find the inverse, we need to solve for in terms of where .
- Writing the Function as an Equation: Write the function as an equation with .Let , so we have .
- Swapping and : Swap and to begin finding the inverse function.We replace with and with to get .
- Solving for y: Solve for y to find the inverse function.We need to isolate y on one side of the equation. Start by dividing both sides by .
- Writing the Inverse Function: Continue solving for .Add to both sides of the equation to isolate .
- Writing the Inverse Function: Continue solving for .Add to both sides of the equation to isolate . Write the inverse function.Now that we have by itself, we can write the inverse function as .