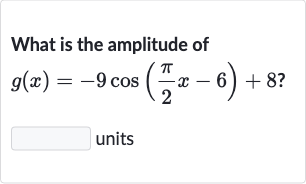Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

What is the amplitude of

g(x)=-9cos((pi)/(2)x-6)+8?
units

What is the amplitude of $\newline$ $g(x)=-9 \cos \left(\frac{\pi}{2} x-6\right)+8 ?$ $\newline$ units

View step-by-step help

View step-by-step help

Solve equations with variables on both sides: fractional coefficients

Full solution

Q. What is the amplitude of

\newline

g(x)=-9 \cos \left(\frac{\pi}{2} x-6\right)+8 ?

\newline

units

Identify Amplitude Coefficient: The amplitude of a cosine function is the absolute value of the coefficient in front of the cosine term. In the function $g(x) = -9\cos\left(\frac{\pi}{2}x - 6\right) + 8$ , the coefficient in front of the cosine term is $-9$ .
Calculate Absolute Value: To find the amplitude, we take the absolute value of $-9$ , which is $9$ .
Amplitude Determination: The amplitude does not depend on the horizontal shift (in this case, $-6$ ) or the vertical shift (in this case, $+8$ ). It is solely determined by the coefficient of the cosine term.
Final Amplitude Calculation: Therefore, the amplitude of the function $g(x)$ is $9$ units.

More problems from Solve equations with variables on both sides: fractional coefficients

Question

Write the sentence as an equation.

\newline

138

is equal to the quotient of

w

30

\newline

/

) if you want to use a division sign.

\newline

Posted 3 months ago

Question

\newline

(\frac{2}{7})x= -14

\newline

x =

Posted 4 months ago

Question

(\frac{8}{5})x= 32

Posted 4 months ago

Question

\newline

2+2

\newline

Posted 4 days ago

Question

-27x+18y=54

Posted 4 months ago

Question

\newline

48.5-0.434=

Posted 4 months ago

Question

\newline

96.7-0.163=

Posted 4 months ago

Question

\newline

4.43-0.283=

Posted 4 months ago

Question

\newline

3.05-0.338=

Posted 4 months ago

Question

\newline

6.168-0.432=

Posted 4 months ago