Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

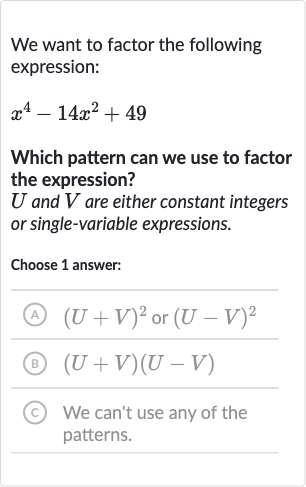
We want to factor the following expression:

x^(4)-14x^(2)+49
Which pattern can we use to factor the expression?

U and
V are either constant integers or single-variable expressions.
Choose 1 answer:
(A)
(U+V)^(2) or
(U-V)^(2)
(B)
(U+V)(U-V)
(c) We can't use any of the patterns.

We want to factor the following expression: $\newline$ $x^{4}-14 x^{2}+49$ $\newline$ Which pattern can we use to factor the expression? $\newline$ $U$ and $V$ are either constant integers or single-variable expressions. $\newline$ Choose $1$ answer: $\newline$ (A) $(U+V)^{2}$ or $(U-V)^{2}$ $\newline$ (B) $(U+V)(U-V)$ $\newline$ (C) We can't use any of the patterns.

View step-by-step help

View step-by-step help

Mean, median, mode, and range: find the missing number

Full solution

Q. We want to factor the following expression:

\newline

x^{4}-14 x^{2}+49

\newline

Which pattern can we use to factor the expression?

\newline

U

and

V

are either constant integers or single-variable expressions.

\newline

Choose

1

answer:

\newline

(A)

(U+V)^{2}

or

(U-V)^{2}

\newline

(B)

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Rephrasing the problem: First, let's rephrase the ＂What pattern can be used to factor the expression $x^4 - 14x^2 + 49$ ?＂
Identifying the structure: Identify the structure of the given expression: $x^4 - 14x^2 + 49$ . Notice that it resembles the structure of a perfect square trinomial, which is $a^2 - 2ab + b^2$ or $a^2 + 2ab + b^2$ , where the first and last terms are perfect squares and the middle term is twice the product of the square roots of the first and last terms.
Checking for perfect squares: Determine if the first term $x^4$ and the last term $49$ are perfect squares. The first term $x^4$ is a perfect square because $(x^2)^2 = x^4$ . The last term $49$ is a perfect square because $7^2 = 49$ .
Verifying the middle term: Check if the middle term $-14x^2$ fits the pattern of twice the product of the square roots of the first and last terms. The square root of $x^4$ is $x^2$ , and the square root of $49$ is $7$ , so twice the product of $x^2$ and $7$ is $2 \times x^2 \times 7 = 14x^2$ . Since the middle term is $-14x^2$ , it fits the pattern of a perfect square trinomial with a negative middle term, which corresponds to $(a - b)^2$ .
Writing as a perfect square trinomial: Write the expression as a perfect square trinomial using the pattern $(a - b)^2$ , where $a$ is the square root of the first term and $b$ is the square root of the last term. In this case, $a = x^2$ and $b = 7$ , so the factored form is $(x^2 - 7)^2$ .

More problems from Mean, median, mode, and range: find the missing number

Question

J

(-4,4)

\newline

Select all of the following that are

4

units from point

J

\newline

Choose all answers that apply:

\newline

x

\newline

(-4,8)

\newline

y

Posted 3 months ago

Question

Here are four matrices:

\newline

\begin{array}{l} \mathbf{A}=\left[\begin{array}{l} 3 \\ 4 \\ 5 \end{array}\right] \quad \mathbf{B}=\left[\begin{array}{cc} -1 & -3 \\ 0 & 4 \end{array}\right] \\ \mathbf{C}=\left[\begin{array}{l} 2 \\ 1 \\ 0 \end{array}\right] \quad \mathbf{D}=\left[\begin{array}{ll} -5 & 2 \\ 3 & -4 \end{array}\right] \end{array}

\newline

Which of the following calculations are possible?

\newline

Choose all answers that apply:

\newline

\mathbf{A}+\mathbf{C}

\newline

\mathbf{B}+\mathbf{C}

\newline

\mathbf{C}-\mathbf{A}

\newline

\mathbf{D}-\mathbf{B}

Posted 3 months ago

Question

What is the median?

\newline

4, 8, -4, 2, 1

\newline

Posted 2 months ago

Question

What is the mode?

\newline

-4, -6, -10, -4, -6, -4, -10, -4

\newline

Posted 2 months ago

Question

Select the outlier in the data set.

\newline

50, 60, 68, 86, 87, 91, 99, 347

\newline

If the outlier were removed from the data set, would the mean increase or decrease?

\newline

\newline

\newline

Posted 2 months ago

Question

Select the outlier in the data set.

\newline

8, 75, 79, 84, 85, 92, 94, 96

\newline

If the outlier were removed from the data set, would the mean increase or decrease?

\newline

\newline

\newline

Posted 2 months ago

Question

Select the outlier in the data set.

\newline

6, 62, 80, 82, 84, 88, 92, 99

\newline

If the outlier were removed from the data set, would the mean increase or decrease?

\newline

\newline

\newline

Posted 2 months ago

Question

Select the outlier in the data set.

\newline

6, 75, 77, 84, 86, 93, 97, 99

\newline

If the outlier were removed from the data set, would the mean increase or decrease?

\newline

\newline

\newline

Posted 2 months ago

Question

Select the outlier in the data set.

\newline

8, 62, 66, 73, 77, 80, 84, 93

\newline

If the outlier were removed from the data set, would the mean increase or decrease?

\newline

\newline

\newline

Posted 2 months ago

Question

Plot the vertex. Then plot another point on the parabola. If you make a mistake, you can erase your parabola by selecting the second point and placing it on top of the first.

\newline

\newline

Posted 7 hours ago