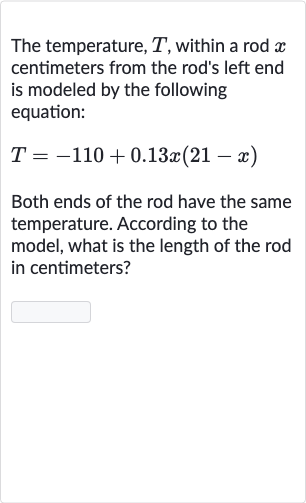
The temperature, T, within a rod x centimeters from the rod's left end is modeled by the following equation: T=-110+0.13 x(21-x) Both ends of the rod have the same temperature. According to the model, what is the length of the rod in centimeters?

Question

The temperature,  T, within a rod  x centimeters from the rod's left end is modeled by the following equation:  T=-110+0.13 x(21-x) Both ends of the rod have the same temperature. According to the model, what is the length of the rod in centimeters?

Bytelearn · Accepted Answer

Temperature Model: We are given the temperature model for a rod: T = -110 + 0.13x(21 - x) We need to find the length of the rod, which means we need to find the value of x when T is the same at both ends of the rod. Since both ends of the rod have the same temperature, we can assume that the temperature is at its extreme (either maximum or minimum) at the center of the rod. This is because the temperature distribution is typically symmetrical in such problems. To find the length, we need to find the value of x at both ends of the rod.Find Critical Points: Let's find the critical points of the temperature function by taking the derivative and setting it to zero. This will give us the x-value where the temperature is at an extreme, which should correspond to the center of the rod if the temperature is symmetrical. dT/dx = 0.13(21 - 2x) Set dT/dx to zero and solve for x: 0 = 0.13(21 - 2x) 0 = 2.73 - 0.26x 0.26x = 2.73 x = 2.73 / 0.26 x = 10.5Calculate Length: The value x = 10.5 cm represents the position of the extreme temperature along the rod, which should be the center if the temperature distribution is symmetrical. Therefore, the total length of the rod is twice this value. Length of the rod = 2 * 10.5 cm Length of the rod = 21 cm

The temperature, $T$ , within a rod $x$ centimeters from the rod's left end is modeled by the following equation: $\newline$ $T=-110+0.13 x(21-x)$ $\newline$ Both ends of the rod have the same temperature. According to the model, what is the length of the rod in centimeters?

Full solution

More problems from Scale drawings: word problems

Related topics