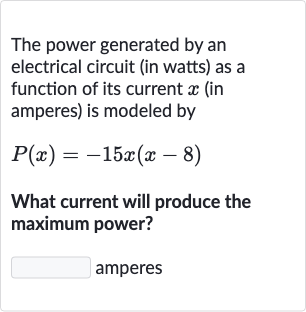
The power generated by an electrical circuit (in watts) as a function of its current x (in amperes) is modeled by P(x)=-15 x(x-8) What current will produce the maximum power? amperes

Question

The power generated by an electrical circuit (in watts) as a function of its current  x (in amperes) is modeled by  P(x)=-15 x(x-8) What current will produce the maximum power? amperes

Bytelearn · Accepted Answer

Find Parabola Vertex: To find the current that will produce the maximum power, we need to find the vertex of the parabola represented by the quadratic function P(x) = -15x(x - 8). Since the coefficient of x^2 is negative (-15), the parabola opens downwards, and the vertex will give us the maximum point.Rewrite Quadratic Function: The quadratic function is in the form P(x) = ax^2 + bx + c. To find the x-coordinate of the vertex, we use the formula -b/(2a). First, we need to rewrite the function in standard form. P(x) = -15x^2 + 120x Here, a = -15 and b = 120.Calculate x-coordinate: Now we apply the formula to find the x-coordinate of the vertex: x = -b/(2a) = -120/(2 * -15) = -120 / -30 = 4Verify Calculations: The x-coordinate of the vertex is 4 amperes. This is the current that will produce the maximum power in the electrical circuit.To ensure there are no math errors, we can check our calculations: -15 * 4 * (4 - 8) = -15 * 4 * -4 = 240 This is a positive value, and since the parabola opens downwards, it confirms that the vertex is indeed a maximum point.

The power generated by an electrical circuit (in watts) as a function of its current $x$ (in amperes) is modeled by $\newline$ $P(x)=-15x(x-8)$ $\newline$ What current will produce the maximum power? $\newline$ amperes

Full solution

More problems from Find the roots of factored polynomials

Related topics

The power generated by an electrical circuit (in watts) as a function of its current xxx (in amperes) is modeled by\newlineP(x)=−15x(x−8)P(x)=-15x(x-8)P(x)=−15x(x−8)\newlineWhat current will produce the maximum power?\newlineamperes

The power generated by an electrical circuit (in watts) as a function of its current $x$ (in amperes) is modeled by $\newline$ $P(x)=-15x(x-8)$ $\newline$ What current will produce the maximum power? $\newline$ amperes