Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

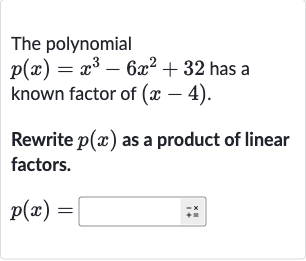
The polynomial p(x)=x^(3)-6x^(2)+32 has a known factor of (x-4). Rewrite p(x) as a product of linear factors. p(x)=

The polynomial p(x)=x3−6x2+32 p(x)=x^{3}-6 x^{2}+32 has a known factor of (x−4) (x-4) .\newlineRewrite p(x) p(x) as a product of linear factors.\newlinep(x)= p(x)=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Find the inverse of a linear functionright chevron icon

Full solution

Q. The polynomial p(x)=x3−6x2+32 p(x)=x^{3}-6 x^{2}+32 has a known factor of (x−4) (x-4) .\newlineRewrite p(x) p(x) as a product of linear factors.\newlinep(x)= p(x)=
  1. Perform polynomial division: Given that (x−4)(x-4) is a known factor of p(x)p(x), we will perform polynomial division to divide p(x)p(x) by (x−4)(x-4). Reasoning: To find the other factors, we need to divide the polynomial by the known factor. Calculation: Perform the division of p(x)p(x) by (x−4)(x-4). Math error check:
  2. Set up the division: Set up the division of p(x)p(x) by (x−4)(x-4).\newlineReasoning: We will use either long division or synthetic division to divide the polynomial.\newlineCalculation: \newlinep(x)=x3−6x2+0x+32p(x) = x^3 - 6x^2 + 0x + 32 (Note: The term 0x0x is added for the missing x-term)\newlineDivide by (x−4)(x-4).\newlineMath error check:
  3. Perform the division: Perform the division.\newlineReasoning: By dividing, we will find the quotient which will give us the other factors of p(x)p(x).\newlineCalculation: \newlineWhen we divide x3−6x2+0x+32x^3 - 6x^2 + 0x + 32 by (x−4)(x-4), we get x2−2x−8x^2 - 2x - 8 as the quotient.\newlineMath error check:
  4. Factor the quotient: Factor the quotient x2−2x−8x^2 - 2x - 8. Reasoning: The quotient is a quadratic polynomial, which can be factored into linear factors if it has real roots. Calculation: x2−2x−8=(x−4)(x+2)x^2 - 2x - 8 = (x - 4)(x + 2) Math error check:
  5. Write p(x)p(x) as a product: Write p(x)p(x) as a product of linear factors.\newlineReasoning: Since we have factored the quotient and we know (x−4)(x-4) is a factor, we can write p(x)p(x) as the product of these factors.\newlineCalculation: \newlinep(x)=(x−4)(x−4)(x+2)p(x) = (x - 4)(x - 4)(x + 2)\newlineMath error check:

More problems from Find the inverse of a linear function

Question
Find the inverse of the function. y=x+3 y = x + 3 Write your answer in the form ax+b ax + b . Simplify any fractions. y= y = _____
Get tutor helpright-arrow

Posted 5 months ago

Question
Find (r∘s)(x)(r \circ s)(x).\newliner(x) = 33x\newlines(x) = 22x + 55\newlineWrite your answer as a polynomial in simplest form.\newline(r∘s)(x)=(r \circ s)(x) = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
What is (f+g)(x)(f + g)(x)?\newlinef(x)=−3xf(x) = -3x\newlineg(x)=3x+1g(x) = 3x + 1\newline\newlineWrite your answer as a polynomial or a rational function in simplest form.\newline‾\underline{\hspace{3cm}}\newline
Get tutor helpright-arrow

Posted 7 months ago

Question
What is (f∗g)(x)(f * g)(x)?\newlinef(x)=−x+4f(x) = -x + 4\newlineg(x)=4xg(x) = 4x\newline\newlineWrite your answer as a polynomial or a rational function in simplest form.\newline‾\underline{\hspace{3em}}\newline
Get tutor helpright-arrow

Posted 7 months ago

Question
What is the domain of (fg)(x)\left(\frac{f}{g}\right)(x)?\newlinef(x)=2xf(x) = 2x\newlineg(x)=−5x+3g(x) = -5x + 3\newlineChoices:\newline[A]All real numbers[\text{A]All real numbers}\newline[B]All real numbers except 35[\text{B]All real numbers except } \frac{3}{5}\newline[C]All real numbers except −35[\text{C]All real numbers except } -\frac{3}{5}\newline[D]All real numbers except 53[\text{D]All real numbers except } \frac{5}{3}
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the inverse of the function.\newliney = −3x-3x \newlineWrite your answer in the form ax+bax + b. Simplify any fractions.\newliney = ______
Get tutor helpright-arrow

Posted 7 months ago

Question
Find (f∘g)(0)(f \circ g)(0).\newlinef(x)=6xf(x) = 6x\newlineg(x)=x2+4xg(x) = x^2 + 4x\newline(f∘g)(0)=(f \circ g)(0) = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
Find (f∘g)(x)(f \circ g)(x).\newlinef(x)=x2+5f(x) = x^2 + 5\newlineg(x)=4xg(x) = 4x\newlineWrite your answer as a polynomial in simplest form.\newline(f∘g)(x)=(f \circ g)(x) = ______
Get tutor helpright-arrow

Posted 7 months ago

Question
Is f(x) f(x) the inverse function of g(x) g(x) ?\newlinef(x)=x f(x) = x \newlineg(x)=−4x g(x) = -4x \newlineChoices:\newline[yes] \text{[yes]} \newline[no] \text{[no]}
Get tutor helpright-arrow

Posted 7 months ago

Question
What is (f∗g)(x)(f * g)(x)? f(x)=3x2+9xf(x) = 3x^2 + 9x g(x)=3xg(x) = 3x Write your answer as a polynomial or a rational function in simplest form. ______
Get tutor helpright-arrow

Posted 5 months ago

View all (120)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant