Full solution
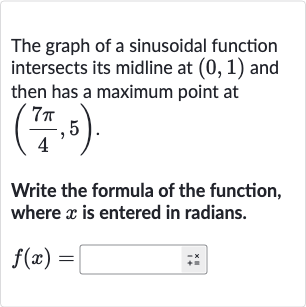
Q. The graph of a sinusoidal function intersects its midline at and then has a maximum point at .Write the formula of the function, where is entered in radians.
- Determine Amplitude: Determine the amplitude of the sinusoidal function.The amplitude is the distance from the midline to the maximum point. Since the midline is at and the maximum point is at , the amplitude is .
- Determine Vertical Shift: Determine the vertical shift . The midline of the function is also the vertical shift . Since the function intersects the midline at , is .
- Determine Period: Determine the period of the sinusoidal function.The period is the distance between two consecutive maximum points. Since we only have one maximum point, we can use the fact that the maximum point occurs at and the function intersects the midline at . The next intersection with the midline after a maximum would be a quarter period away, so the period is times the -coordinate of the maximum point, which is .
- Determine Value of B: Determine the value of B.The value of B is related to the period by the formula . Substituting the period , we get .
- Determine Phase Shift: Determine the phase shift . Since the function intersects its midline at , there is no horizontal shift, so .
- Write Sinusoidal Function: Write the equation of the sinusoidal function.We have found:Substitute these values into the general form of the sinusoidal function .
More problems from Write equations of cosine functions using properties
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help