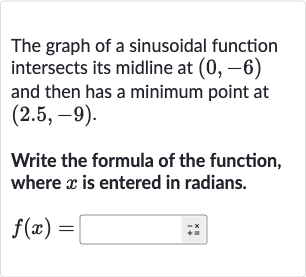
The graph of a sinusoidal function intersects its midline at (0,-6) and then has a minimum point at (2.5,-9). Write the formula of the function, where x is entered in radians. f(x)=◻

Question

The graph of a sinusoidal function intersects its midline at  (0,-6) and then has a minimum point at  (2.5,-9). Write the formula of the function, where  x is entered in radians.  f(x)=◻

Bytelearn · Accepted Answer

Midline and Vertical Shift: The midline of the sinusoidal function is the horizontal line that passes through the average of the maximum and minimum values of the function. Since the function intersects the midline at (0,-6), this is the vertical shift, D, of the function.Amplitude Calculation: The minimum point of the function is at (2.5,-9). Since the midline is at -6 and the minimum is at -9, the amplitude, A, of the function is the distance from the midline to the minimum, which is 9 - 6 = 3.Phase Shift Determination: The minimum point occurs at x = 2.5. For a sinusoidal function in the form f(x) = A sin(Bx + C) + D or f(x) = A cos(Bx + C) + D, the minimum point of a cosine function occurs at x = π/B (for the first minimum after the phase shift). However, since we have a sine function and the minimum occurs at x = 2.5, we can deduce that the phase shift, C, must be such that the sine function reaches its minimum at this point. Since the sine function has a period of 2π, and the minimum point is at 2.5, we can infer that the period must be 2 * 2.5 = 5 radians, because the minimum of a sine function occurs halfway through its period. Therefore, the period, T, is 5 radians, and B = 2π/T = 2π/5.Solving for C: Since the minimum point of a sine function is at π - C/B, and we know that the minimum occurs at x = 2.5, we can set up the equation π - C/(2π/5) = 2.5. Solving for C, we get C = π - (2.5 * 2π/5) = π - π = 0. Therefore, there is no horizontal phase shift, and C = 0.Final Sinusoidal Function: We now have all the parameters for the sinusoidal function: A = 3, B = 2π/5, C = 0, and D = -6. The function is a sine function because it reaches a minimum at x = 2.5, which is not typical for a cosine function without a phase shift. Therefore, the equation of the function is f(x) = 3sin((2π/5)x) - 6.

The graph of a sinusoidal function intersects its midline at $(0,-6)$ and then has a minimum point at $(2.5,-9)$ . $\newline$ Write the formula of the function, where $x$ is entered in radians. $\newline$ $f(x)=\square$

Full solution

More problems from Write equations of cosine functions using properties

Related topics

The graph of a sinusoidal function intersects its midline at (0,−6) (0,-6) (0,−6) and then has a minimum point at (2.5,−9) (2.5,-9) (2.5,−9).\newlineWrite the formula of the function, where x x x is entered in radians.\newlinef(x)=□ f(x)=\square f(x)=□

The graph of a sinusoidal function intersects its midline at $(0,-6)$ and then has a minimum point at $(2.5,-9)$ . $\newline$ Write the formula of the function, where $x$ is entered in radians. $\newline$ $f(x)=\square$