AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
The function is given in three equivalent forms.Which form most quickly reveals the zeros (or roots) of the function?Choose answer:(A) (B) (C) Write one of the zeros.
Full solution
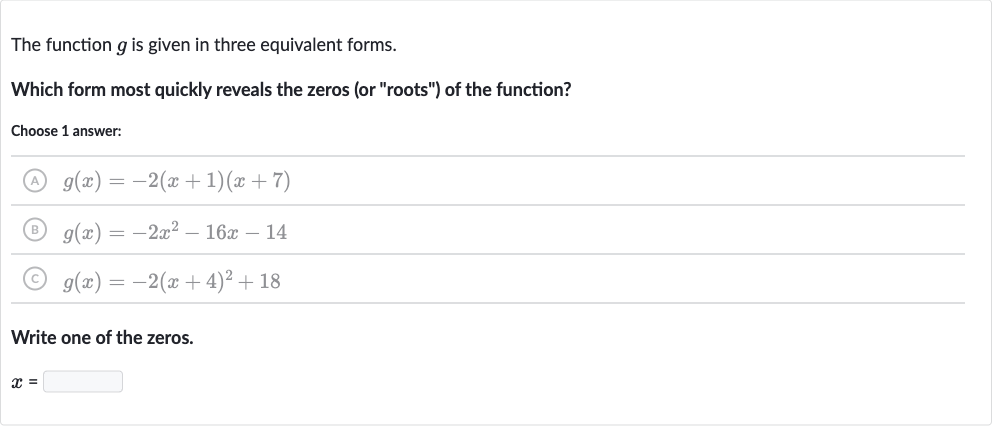
Q. The function is given in three equivalent forms.Which form most quickly reveals the zeros (or roots) of the function?Choose answer:(A) (B) (C) Write one of the zeros.
- Identify Factored Form: To determine which form of the function most quickly reveals the zeros, we need to look for the form that is already factored. The zeros of a function are the values of that make the function equal to zero. In a factored form, we can easily set each factor equal to zero and solve for .
- Analyze Option (A): Option (A) presents the function in factored form: . This form immediately shows the zeros of the function, which are the values of that make each factor zero. In this case, the zeros are and .
- Consider Option (B): Option (B) presents the function in standard quadratic form: . To find the zeros from this form, we would need to factor the quadratic or use the quadratic formula, which is more time-consuming than reading the zeros directly from a factored form.
- Evaluate Option (C: Option (C) presents the function in vertex form: . While this form quickly reveals the vertex of the parabola, it does not immediately show the zeros. We would need to set the function equal to zero and solve for , which is less direct than using a factored form.
- Compare and Choose: Comparing the three forms, we can see that option (A) most quickly reveals the zeros of the function because it is already in factored form. We can write one of the zeros as .
