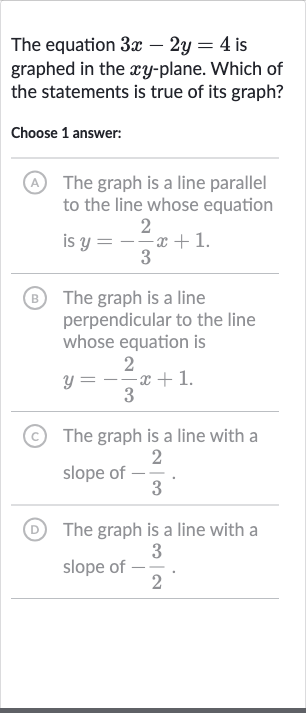
The equation 3x-2y=4 is graphed in the xy-plane. Which of the statements is true of its graph? Choose 1 answer: A The graph is a line parallel to the line whose equation is y=-(2)/(3)x+1. B The graph is a line perpendicular to the line whose equation is y=-(2)/(3)x+1". " (c) The graph is a line with a slope of -(2)/(3). (D) The graph is a line with a slope of -(3)/(2).

Question

The equation  3x-2y=4 is graphed in the  xy-plane. Which of the statements is true of its graph? Choose 1 answer: A The graph is a line parallel to the line whose equation is  y=-(2)/(3)x+1. B The graph is a line perpendicular to the line whose equation is  y=-(2)/(3)x+1". " (c) The graph is a line with a slope of  -(2)/(3). (D) The graph is a line with a slope of  -(3)/(2).

Bytelearn · Accepted Answer

Rewrite Equation: First, let's rewrite the given equation 3x - 2y = 4 in slope-intercept form, which is y = mx + b, where m is the slope and b is the y-intercept. To do this, we solve for y: 3x - 2y = 4 -2y = -3x + 4 y = (3/2)x - 2Identify Slope: Now that we have the equation in slope-intercept form, we can identify the slope of the line. The slope is the coefficient of x, which in this case is 3/2.Compare Slopes: Let's compare the slope of our line with the slopes given in the answer choices: A) The line y = -(2/3)x + 1 has a slope of -(2/3). Our line has a slope of 3/2, which is not the same, so this statement is false. B) To be perpendicular, the slopes of two lines must be negative reciprocals of each other. The negative reciprocal of 3/2 is -2/3, which matches the slope of the line in choice B. Therefore, this statement is true. C) The graph of our line does not have a slope of -(2/3), so this statement is false. D) The graph of our line does not have a slope of -(3/2), so this statement is false.

Full solution

More problems from Write an equation for a parallel or perpendicular line

Related topics