AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
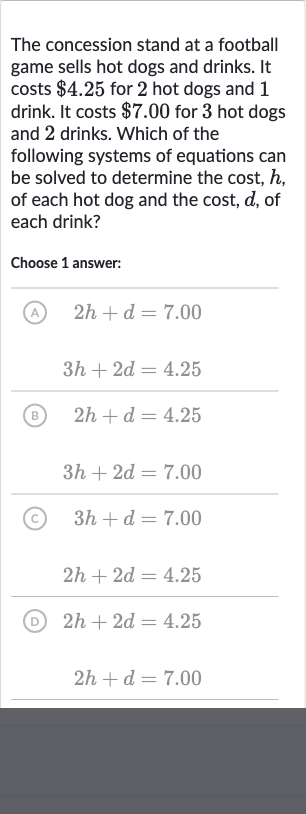
The concession stand at a football game sells hot dogs and drinks. It costs for hot dogs and drink. It costs for hot dogs and drinks. Which of the following systems of equations can be solved to determine the cost, , of each hot dog and the cost, , of each drink?Choose answer:(A) (B) (C) (D)
Full solution
Q. The concession stand at a football game sells hot dogs and drinks. It costs for hot dogs and drink. It costs for hot dogs and drinks. Which of the following systems of equations can be solved to determine the cost, , of each hot dog and the cost, , of each drink?Choose answer:(A) (B) (C) (D)
- Define Variables: Let's denote the cost of each hot dog as and the cost of each drink as . We are given two scenarios with their total costs. We need to translate these scenarios into two equations to form a system of equations.
- Translate Scenarios to Equations: For the first scenario, we have hot dogs and drink costing . This can be represented by the equation .
- Match Equations to Answer Choices: For the second scenario, we have hot dogs and drinks costing . This can be represented by the equation .
- Identify Correct Answer Choice: Now, let's match our equations to the answer choices. We have the equations:. . We need to find the choice that matches these equations.
- Identify Correct Answer Choice: Now, let's match our equations to the answer choices. We have the equations:. . We need to find the choice that matches these equations.Looking at the answer choices, we can see that choice (B) matches our equations:(B)
- Identify Correct Answer Choice: Now, let's match our equations to the answer choices. We have the equations:. . We need to find the choice that matches these equations.Looking at the answer choices, we can see that choice (B) matches our equations:(B) Therefore, the correct system of equations to determine the cost of each hot dog () and the cost of each drink () is given by choice (B).
More problems from Counting principle
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
