Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Solve for
x.

-9x+5 < 17quad AND
quad13 x+25 < -1
Choose 1 answer:
(A)
x < -2 or
x > -(4)/(3)
(B)
x < -2
(C)
x > -(4)/(3)
(D) There are no solutions
(E) All values of
x are solutions

Solve for $x$ . $\newline$ -9 x+5<17 \quad AND \quad 13 x+25<-1 $\newline$ Choose $1$ answer: $\newline$ (A) x<-2 or x>-\frac{4}{3} $\newline$ (B) x<-2 $\newline$ (C) x>-\frac{4}{3} $\newline$ (D) There are no solutions $\newline$ (E) All values of $x$ are solutions

View step-by-step help

View step-by-step help

Is (x, y) a solution to the system of linear inequalities?

Full solution

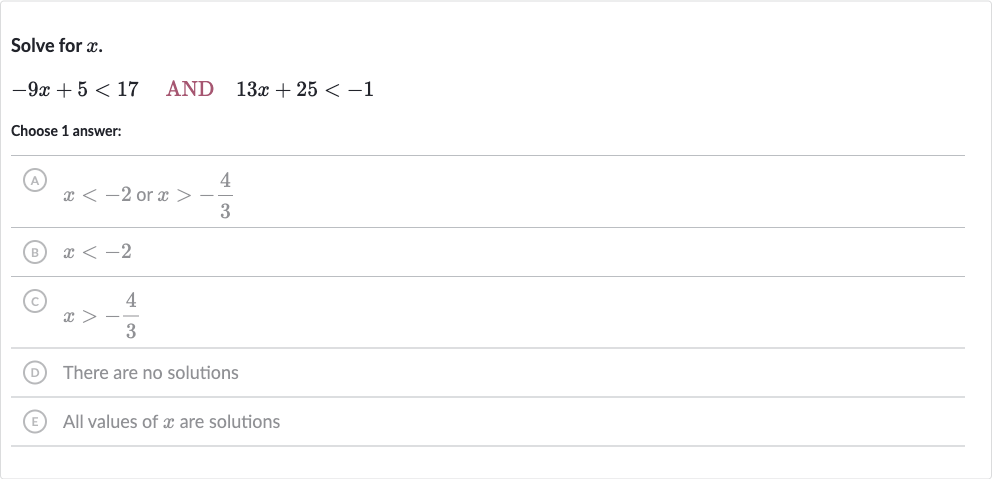
Q. Solve for

x

.

\newline

-9 x+5<17 \quad

AND

\quad 13 x+25<-1

\newline

Choose

1

answer:

\newline

(A)

x<-2

or

x>-\frac{4}{3}

\newline

(B)

x<-2

\newline

(C)

x>-\frac{4}{3}

\newline

(D) There are no solutions

\newline

(E) All values of

x

are solutions

Solve first inequality: First, let's solve the inequality -9x + 5 < 17. $\newline$ Subtract $5$ from both sides to isolate the term with $x$ . $\newline$ -9x + 5 - 5 < 17 - 5 $\newline$ -9x < 12 $\newline$ Now, divide both sides by $-9$ , remembering to reverse the inequality sign because we are dividing by a negative number. $\newline$ x > -\frac{12}{-9} $\newline$ x > \frac{4}{3}
Solve second inequality: Next, let's solve the second inequality 13x + 25 < -1. $\newline$ Subtract $25$ from both sides to isolate the term with $x$ . $\newline$ 13x + 25 - 25 < -1 - 25 $\newline$ 13x < -26 $\newline$ Now, divide both sides by $13$ to solve for $x$ . $\newline$ x < -26 / 13 $\newline$ x < -2
Combine inequalities and find solution: Now we have two inequalities to combine into one solution set: $\newline$ x > \frac{4}{3} and x < -2. $\newline$ However, these two inequalities do not overlap; there is no number that is both greater than $\frac{4}{3}$ and less than $-2$ . Therefore, there is no solution to the system of inequalities.

More problems from Is (x, y) a solution to the system of linear inequalities?

Question

Write the following inequality in slope-intercept form.

\newline

9x+y \geq -17

\newline

Write your answer with

y

first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.

\newline

Posted 5 months ago

Question

(2,\,1)

make the inequality

2x + 15y < 20

\newline

\newline

\newline

Posted 5 months ago

Question

Jack is fixing up his home and must spend less than

\$4,800

to hire carpenters and painters. Carpenters charge

\$60

per hour and painters charge

\$32

\newline

Select the inequality in standard form that describes this situation. Use the given numbers and the following variables.

\newline

x =

the number of hours of carpentry

\newline

y =

the number of hours of painting

\newline

\newline

32x + 60y < 4,800

\newline

60x + 32y < 4,800

\newline

32x - 60y < 4,800

\newline

60x - 32y < 4,800

Posted 5 months ago

Question

(2,\, 10)

a solution to this system of inequalities?

\newline

y \geq x + 10

\newline

y \leq 9x + 5

\newline

\newline

\newline

Posted 5 months ago

Question

Write the following inequality in slope-intercept form.

\newline

4x - 2y \leq 8

\newline

Write your answer with

y

first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.

Posted 9 months ago

Question

Write the following inequality in slope-intercept form.

\newline

-3x + 5y > 15

\newline

Write your answer with

y

first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.

Posted 9 months ago

Question

Write the following inequality in slope-intercept form.

\newline

2x + 3y < -6

\newline

Write your answer with

y

first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.

Posted 9 months ago

Question

Write the following inequality in slope-intercept form.

\newline

-7x - y \geq 21

\newline

Write your answer with

y

first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.

Posted 8 months ago

Question

Write the following inequality in slope-intercept form.

\newline

5x - 4y > -20

\newline

Write your answer with

y

first, followed by an inequality symbol. Use integers, proper fractions, and improper fractions in simplest form.

Posted 9 months ago

Question

Miyoko's goal is to earn more than

(950

this week. She earns

)250

for every day she works as a cryptographer, and earns

\$180

for every day she works as a geologist.

\newline

Write an inequality that represents the number of days Miyoko should work as a cryptographer

C

and as a geologist

G

to achieve her goal.

Posted 10 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant