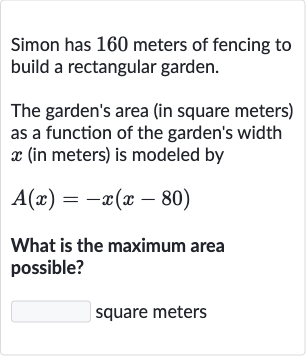
Simon has 160 meters of fencing to build a rectangular garden. The garden's area (in square meters) as a function of the garden's width x (in meters) is modeled by A(x)=-x(x-80) What is the maximum area possible? square meters

Question

Simon has 160 meters of fencing to build a rectangular garden. The garden's area (in square meters) as a function of the garden's width  x (in meters) is modeled by  A(x)=-x(x-80) What is the maximum area possible? square meters

Bytelearn · Accepted Answer

Given Function: We are given the function A(x) = -x(x - 80), which represents the area of the garden in terms of its width x. To find the maximum area, we need to find the vertex of the parabola represented by this quadratic function. Since the coefficient of x^2 is negative, the parabola opens downwards, and the vertex will give us the maximum area.Find Vertex: The quadratic function is in the form A(x) = ax^2 + bx + c. To find the x-coordinate of the vertex, we use the formula -b/(2a). In our function, a = -1 and b = 80, so we plug these values into the formula.Calculate x-coordinate: Calculating the x-coordinate of the vertex: x = -b/(2a) = -80/(2 * -1) = -80/(-2) = 40.Find Maximum Area: Now that we have the x-coordinate of the vertex, we can find the maximum area by plugging x = 40 back into the function A(x).Calculate Maximum Area: Calculating the maximum area: A(40) = -40(40 - 80) = -40(-40) = 1600 square meters.Final Result: We have found the maximum area of the garden to be 1600 square meters when the width x is 40 meters.

Simon has $160$ meters of fencing to build a rectangular garden. $\newline$ The garden's area (in square meters) as a function of the garden's width $\newline$ $x$ (in meters) is modeled by $\newline$ $A(x)=-x(x-80)$ $\newline$ What is the maximum area possible? $\newline$ square meters

Full solution

More problems from Find the roots of factored polynomials

Related topics