Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

If
u^(2)-ut=6 and
t^(2)-ut=-2, what is the value of
2(u-t)^(2) ?

If $u^{2}-u t=6$ and $t^{2}-u t=-2$ , what is the value of $2(u-t)^{2}$ ?

View step-by-step help

View step-by-step help

Composition of linear and quadratic functions: find a value

Full solution

Q. If

u^{2}-u t=6

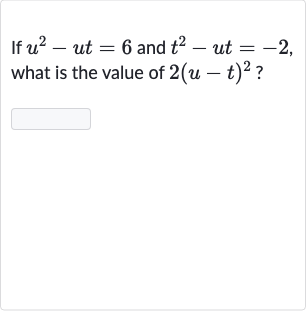
and

t^{2}-u t=-2

, what is the value of

2(u-t)^{2}

?

Given Equations: We are given two equations: $\newline$ $1$ . $u^{2} - ut = 6$ $\newline$ $2$ . $t^{2} - ut = -2$ $\newline$ We need to find the value of $2(u-t)^{2}$ . $\newline$ First, let's express $(u-t)^{2}$ in terms of $u$ and $t$ . $\newline$ $(u-t)^{2} = u^{2} - 2ut + t^{2}$
Express $(u-t)^{2}$ : Now, let's add the two given equations to eliminate the $-ut$ term: $\newline$ $(u^{2} - ut) + (t^{2} - ut) = 6 - 2$ $\newline$ $u^{2} + t^{2} - 2ut = 4$ $\newline$ Notice that $u^{2} + t^{2} - 2ut$ is the expanded form of $(u-t)^{2}$ .
Substitute $(u-t)^{2}$ Term: Since we have found that $u^{2} + t^{2} - 2ut = 4$ , we can say that: $(u-t)^{2} = 4$ Now, we need to find the value of $2(u-t)^{2}$ . $2(u-t)^{2} = 2 \times 4$
Calculate $2(u-t)^{2}$ : Calculate the final value: $\newline$ $2(u-t)^{2} = 2 \times 4 = 8$

More problems from Composition of linear and quadratic functions: find a value

Question

Find the inverse of the function.

y = x + 3

Write your answer in the form

ax + b

. Simplify any fractions.

y =

Posted 5 months ago

Question

(r \circ s)(x)

\newline

3

\newline

2

5

\newline

Write your answer as a polynomial in simplest form.

\newline

(r \circ s)(x) =

Posted 7 months ago

Question

(f + g)(x)

\newline

f(x) = -3x

\newline

g(x) = 3x + 1

\newline

\newline

Write your answer as a polynomial or a rational function in simplest form.

\newline

\underline{\hspace{3cm}}

\newline

Posted 7 months ago

Question

(f * g)(x)

\newline

f(x) = -x + 4

\newline

g(x) = 4x

\newline

\newline

Write your answer as a polynomial or a rational function in simplest form.

\newline

\underline{\hspace{3em}}

\newline

Posted 7 months ago

Question

What is the domain of

\left(\frac{f}{g}\right)(x)

\newline

f(x) = 2x

\newline

g(x) = -5x + 3

\newline

\newline

[\text{A]All real numbers}

\newline

[\text{B]All real numbers except } \frac{3}{5}

\newline

[\text{C]All real numbers except } -\frac{3}{5}

\newline

[\text{D]All real numbers except } \frac{5}{3}

Posted 9 months ago

Question

Find the inverse of the function.

\newline

-3x

\newline

Write your answer in the form

ax + b

. Simplify any fractions.

\newline

Posted 7 months ago

Question

(f \circ g)(0)

\newline

f(x) = 6x

\newline

g(x) = x^2 + 4x

\newline

(f \circ g)(0) =

Posted 7 months ago

Question

(f \circ g)(x)

\newline

f(x) = x^2 + 5

\newline

g(x) = 4x

\newline

Write your answer as a polynomial in simplest form.

\newline

(f \circ g)(x) =

Posted 7 months ago

Question

f(x)

the inverse function of

g(x)

\newline

f(x) = x

\newline

g(x) = -4x

\newline

\newline

\text{[yes]}

\newline

\text{[no]}

Posted 7 months ago

Question

(f * g)(x)

f(x) = 3x^2 + 9x

g(x) = 3x

Write your answer as a polynomial or a rational function in simplest form. ______

Posted 5 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant