Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

For a given input value x, the function f outputs a value y to satisfy the following equation. -5x-4y=-8 Write a formula for f(x) in terms of x. f(x)=

For a given input value xx, the function ff outputs a value yy to satisfy the following equation.\newline−5x−4y=−8-5x-4y=-8\newlineWrite a formula for f(x)f(x) in terms of xx.\newlinef(x)=f(x)=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Find the inverse of a linear functionright chevron icon

Full solution

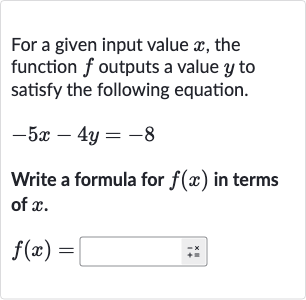
Q. For a given input value xx, the function ff outputs a value yy to satisfy the following equation.\newline−5x−4y=−8-5x-4y=-8\newlineWrite a formula for f(x)f(x) in terms of xx.\newlinef(x)=f(x)=
  1. Isolate variable y: Isolate the variable y in the equation −5x−4y=−8-5x - 4y = -8 to solve for f(x) in terms of x.\newlineWe add 5x5x to both sides of the equation to get −4y=5x−8-4y = 5x - 8.
  2. Divide by ext{−4-4}: Divide both sides of the equation by ext{−4-4} to solve for ext{y}.\newlineDoing this, we get ext{y} = \frac{(55 ext{x} - 88)}{ ext{−4-4}}.
  3. Simplify equation for y: Simplify the equation for y.\newlineWe can simplify the equation to y=−5x4+2y = -\frac{5x}{4} + 2, since dividing both terms by −4-4 gives us y=−54⋅x+84y = -\frac{5}{4} \cdot x + \frac{8}{4}.
  4. Write function f(x): Write the function f(x) in terms of x.\newlineSince y is the output of the function f for the input x, we can write f(x)=−54x+2f(x) = -\frac{5}{4}x + 2.

More problems from Find the inverse of a linear function

Question
Find the inverse of the function. y=x+3 y = x + 3 Write your answer in the form ax+b ax + b . Simplify any fractions. y= y = _____
Get tutor helpright-arrow

Posted 5 months ago

Question
Find (r∘s)(x)(r \circ s)(x).\newliner(x) = 33x\newlines(x) = 22x + 55\newlineWrite your answer as a polynomial in simplest form.\newline(r∘s)(x)=(r \circ s)(x) = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
What is (f+g)(x)(f + g)(x)?\newlinef(x)=−3xf(x) = -3x\newlineg(x)=3x+1g(x) = 3x + 1\newline\newlineWrite your answer as a polynomial or a rational function in simplest form.\newline‾\underline{\hspace{3cm}}\newline
Get tutor helpright-arrow

Posted 7 months ago

Question
What is (f∗g)(x)(f * g)(x)?\newlinef(x)=−x+4f(x) = -x + 4\newlineg(x)=4xg(x) = 4x\newline\newlineWrite your answer as a polynomial or a rational function in simplest form.\newline‾\underline{\hspace{3em}}\newline
Get tutor helpright-arrow

Posted 7 months ago

Question
What is the domain of (fg)(x)\left(\frac{f}{g}\right)(x)?\newlinef(x)=2xf(x) = 2x\newlineg(x)=−5x+3g(x) = -5x + 3\newlineChoices:\newline[A]All real numbers[\text{A]All real numbers}\newline[B]All real numbers except 35[\text{B]All real numbers except } \frac{3}{5}\newline[C]All real numbers except −35[\text{C]All real numbers except } -\frac{3}{5}\newline[D]All real numbers except 53[\text{D]All real numbers except } \frac{5}{3}
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the inverse of the function.\newliney = −3x-3x \newlineWrite your answer in the form ax+bax + b. Simplify any fractions.\newliney = ______
Get tutor helpright-arrow

Posted 7 months ago

Question
Find (f∘g)(0)(f \circ g)(0).\newlinef(x)=6xf(x) = 6x\newlineg(x)=x2+4xg(x) = x^2 + 4x\newline(f∘g)(0)=(f \circ g)(0) = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
Find (f∘g)(x)(f \circ g)(x).\newlinef(x)=x2+5f(x) = x^2 + 5\newlineg(x)=4xg(x) = 4x\newlineWrite your answer as a polynomial in simplest form.\newline(f∘g)(x)=(f \circ g)(x) = ______
Get tutor helpright-arrow

Posted 7 months ago

Question
Is f(x) f(x) the inverse function of g(x) g(x) ?\newlinef(x)=x f(x) = x \newlineg(x)=−4x g(x) = -4x \newlineChoices:\newline[yes] \text{[yes]} \newline[no] \text{[no]}
Get tutor helpright-arrow

Posted 7 months ago

Question
What is (f∗g)(x)(f * g)(x)? f(x)=3x2+9xf(x) = 3x^2 + 9x g(x)=3xg(x) = 3x Write your answer as a polynomial or a rational function in simplest form. ______
Get tutor helpright-arrow

Posted 5 months ago

View all (120)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant