Full solution
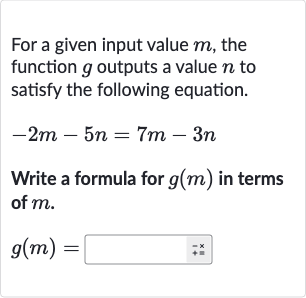
Q. For a given input value , the function outputs a value to satisfy the following equation.Write a formula for in terms of .
- Isolate in equation: First, we need to isolate on one side of the equation to find a formula for in terms of . We start with the given equation:
- Combine like terms: Combine like terms by adding
5 n 5n 2 m 2m m m n n \newline − 2 m + 2 m − 5 n + 5 n = 7 m + 2 m − 3 n + 5 n -2m + 2m - 5n + 5n = 7m + 2m - 3n + 5n \newline \newline 0 m = 9 m + 2 n 0m = 9m + 2n - Subtract
9 m 9m n n 9 m 9m n n 0 m − 9 m = 9 m − 9 m + 2 n 0m - 9m = 9m - 9m + 2n − 9 m = 2 n -9m = 2n - Divide both sides by
2 2 n n 2 2 \newline n = − 9 m 2 n = \frac{-9m}{2} - Formula for g(m): Now we have
n n m m g ( m ) g(m) \newline g ( m ) = − 9 m 2 g(m) = -\frac{9m}{2}
More problems from Find the inverse of a linear function
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help