Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Find the zeros of the function. Enter the solutions from least to greatest.

f(x)=(x-4)^(2)-16
lesser
x=
greater
x=

Find the zeros of the function. Enter the solutions from least to greatest. $\newline$ $f(x)=(x-4)^{2}-16$ $\newline$ lesser $x=$ $\newline$ greater $x=$

View step-by-step help

View step-by-step help

Evaluate exponential functions

Full solution

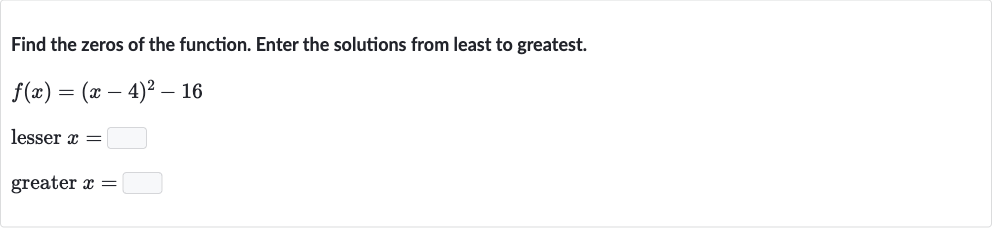
Q. Find the zeros of the function. Enter the solutions from least to greatest.

\newline

f(x)=(x-4)^{2}-16

\newline

lesser

x=

\newline

greater

x=

Find Zeros of the Function: Set the function equal to zero to find its zeros. $\newline$ $f(x) = (x-4)^2 - 16 = 0$
Isolate the Squared Term: Add $16$ to both sides of the equation to isolate the squared term. $\newline$ $(x-4)^2 = 16$
Solve for x: Take the square root of both sides of the equation to solve for x. $\newline$ $\sqrt{(x-4)^2} = \pm\sqrt{16}$ $\newline$ $x - 4 = \pm 4$
Determine the Solutions: Solve for x by adding $4$ to both sides of each equation. $\newline$ For the positive root: $\newline$ $x - 4 + 4 = 4 + 4$ $\newline$ $x = 8$ $\newline$ For the negative root: $\newline$ $x - 4 + 4 = -4 + 4$ $\newline$ $x = 0$
List the Solutions: List the solutions from least to greatest. $\newline$ lesser $x = 0$ $\newline$ greater $x = 8$

More problems from Evaluate exponential functions

Question

x

\newline

5^x=1

\newline

\newline

Write your answer in simplest form.

Posted 9 months ago

Question

Steven opened a savings account and deposited

\$100.00

as principal. The account earns

9\%

interest, compounded annually. What is the balance after

5

\newline

Use the formula

A = P(1 + \frac{r}{n})^{nt}

A

is the balance (final amount),

P

is the principal (starting amount),

r

is the interest rate expressed as a decimal,

n

is the number of times per year that the interest is compounded, and

t

is the time in years.

\newline

Round your answer to the nearest cent.

Posted 9 months ago

Question

Use the following function rule to find

f(1)

\newline

f(x) = 12(7)^x + 8

Posted 9 months ago

Question

The town of Valley View conducted a census this year, which showed that it has a population of

1,800

people. Based on the census data, it is estimated that the population of Valley View will grow by

12\%

\newline

Write an exponential equation in the form

y = a(b)^x

that can model the town population,

y

x

decades after this census was taken.

\newline

Use whole numbers, decimals, or simplified fractions for the values of

a

b

\newline

y =

Posted 1 year ago

Question

x

\newline

7 = 9^x

\newline

Round your answer to the nearest thousandth.

Posted 9 months ago

Question

Solve. Round your answer to the nearest thousandth.

\newline

7 = e^x

\newline

x =

Posted 1 year ago

Question

Solve. Simplify your answer.

\newline

\log u = 1

\newline

u =

Posted 1 year ago

Question

Solve. Simplify your answer.

\newline

7\log x = 7

\newline

x =

Posted 1 year ago

Question

g(t) = 3^t

change over the interval from

t = 3

t = 4

\newline

\newline

g(t)

3

\newline

g(t)

3

\newline

g(t)

3\%

\newline

g(t)

200\%

Posted 9 months ago

Question

f(x)

6

over every unit interval in

x

f(0) = 0

\newline

Which could be a function rule for

f(x)

\newline

\newline

f(x) = 6x

\newline

f(x) = 6^x

\newline

f(x) = \frac{1}{6^x}

\newline

f(x) = \frac{x}{6}

Posted 9 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant