AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
Expand.Your answer should be a polynomial in standard form.
Full solution
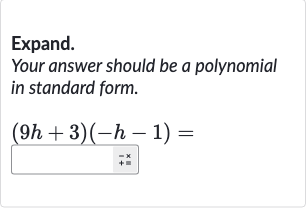
Q. Expand.Your answer should be a polynomial in standard form.
- Apply distributive property: Apply the distributive property to expand the expression .Distribute each term in the first polynomial with each term in the second polynomial .
- Multiply the terms: Multiply the terms from the previous step. (Multiplying by gives ) (Multiplying by gives ) (Multiplying by gives ) (Multiplying by gives )
- Combine like terms: Combine the like terms from the multiplication results.Combine and to get .
- Write final answer: Write the final answer in standard form, which is the form of a polynomial arranged by descending powers of .The final answer is .
