AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
Expand.Your answer should be a polynomial in standard form. =
Full solution
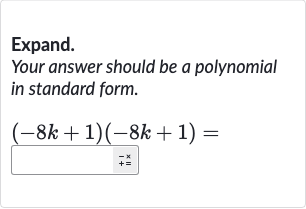
Q. Expand.Your answer should be a polynomial in standard form. =
- Apply distributive property: Apply the distributive property (also known as the FOIL method for binomials) to expand the expression .(\(-8k+)(k+) = (k)(k) + (k)() + ()(k) + ()()
- Multiply first terms: Multiply the first terms .
- Multiply outer terms: Multiply the outer terms .
- Multiply inner terms: Multiply the inner terms .
- Multiply last terms: Multiply the last terms .
- Combine like terms: Combine like terms from the results of steps , , , and .
