Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Expand.
Your answer should be a polynomial in standard form.

(-5d+8)(d-3)=

Expand. $\newline$ Your answer should be a polynomial in standard form. $\newline$ $(-5d+8)(d-3)$ =

View step-by-step help

View step-by-step help

Multiply two binomials

Full solution

Q. Expand.

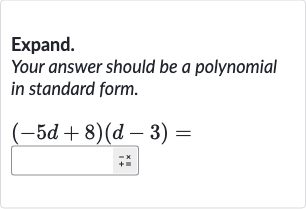
\newline

Your answer should be a polynomial in standard form.

\newline

(-5d+8)(d-3)

=

Apply distributive property: Apply the distributive property to expand the expression $(-5d+8)(d-3)$ . $\newline$ We will distribute each term in the first polynomial $(-5d+8)$ with each term in the second polynomial $(d-3)$ .
Multiply $-5d$ by $d$ : Multiply $-5d$ by $d$ . $\newline$ $-5d \times d = -5d^2$ $\newline$ This gives us the first term of the expanded polynomial.
Multiply $-5d$ by $-3$ : Multiply $-5d$ by $-3$ . $\newline$ $-5d \times -3 = 15d$ $\newline$ This gives us the second term of the expanded polynomial.
Multiply $8$ by $d$ : Multiply $8$ by $d$ . $\newline$ $8$ \times d = $8$ d $\newline$ This gives us the third term of the expanded polynomial.
Multiply $8$ by $-3$ : Multiply $8$ by $-3$ . $\newline$ $8 \times -3 = -24$ $\newline$ This gives us the fourth term of the expanded polynomial.
Combine terms: Combine all the terms to get the polynomial in standard form. $\newline$ The expanded form is the sum of the terms from steps $2$ to $5$ . $\newline$ $-5d^2 + 15d + 8d - 24$
Combine like terms: Combine like terms $15d$ and $8d$ . $15d + 8d = 23d$ Now we have the simplified expanded polynomial. $-5d^2 + 23d - 24$

More problems from Multiply two binomials

Question

Find the degree of this polynomial.

\newline

`5b^{10} + 3b^3`

\newline

Posted 10 months ago

Question

\newline

(9a + 5) - (2a + 4)

\newline

Posted 10 months ago

Question

Find the product. Simplify your answer.

\newline

-3v^2(v^2 - 9)

\newline

Posted 10 months ago

Question

Find the product. Simplify your answer.

\newline

(v - 3)(4v + 1)

\newline

Posted 10 months ago

Question

Find the square. Simplify your answer.

\newline

(3y + 2)^2

\newline

Posted 10 months ago

Question

Divide. If there is a remainder, include it as a simplified fraction.

\newline

(24t^2 + 36t) \div 6t

\newline

Posted 10 months ago

Question

Is the function

q(x) = x^6 - 9

even, odd, or neither?

\newline

\newline

\text{[[even][odd][neither]]}

Posted 1 year ago

Question

Find the product. Simplify your answer.

\newline

-3q^2(-3q^2 + q)

\newline

Posted 1 year ago

Question

Find the product. Simplify your answer.

\newline

(r + 3)(4r + 2)

\newline

Posted 1 year ago

Question

Find the roots of the factored polynomial.

\newline

(x + 7)(x + 4)

\newline

Write your answer as a list of values separated by commas.

\newline

x =

Posted 1 year ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant