AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
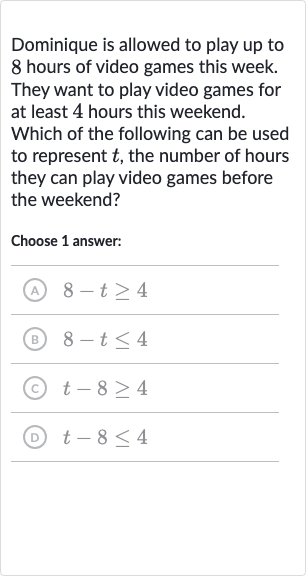
Dominique is allowed to play up to hours of video games this week. They want to play video games for at least hours this weekend.Which of the following can be used to represent , the number of hours they can play video games before the weekend?Choose answer:(A) (B) (C) (D)
Full solution
Q. Dominique is allowed to play up to hours of video games this week. They want to play video games for at least hours this weekend.Which of the following can be used to represent , the number of hours they can play video games before the weekend?Choose answer:(A) (B) (C) (D)
- Total allowed hours: Dominique is allowed to play up to hours of video games for the entire week. They want to ensure that they play at least hours during the weekend. To find the number of hours Dominique can play before the weekend, we need to subtract the hours they plan to play during the weekend from the total allowed hours for the week.
- Inequality representation: Let represent the number of hours Dominique can play video games before the weekend. Since they want to play at least hours during the weekend, the total hours played before the weekend plus the hours played during the weekend should not exceed hours. This can be represented by the inequality:Since they want to play at least hours during the weekend, we can substitute this value into the inequality:
- Isolating in the inequality: To find the expression for , we need to isolate on one side of the inequality. We do this by subtracting from both sides of the inequality:This simplifies to:
- Number of hours before the weekend: The inequality means that the number of hours Dominique can play video games before the weekend is hours or less. This matches option (B) from the given choices.
More problems from Counting principle
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
