Full solution
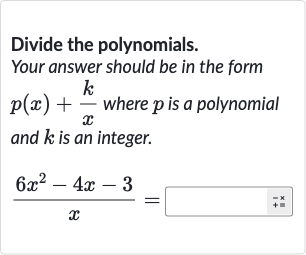
Q. Divide the polynomials.Your answer should be in the form where is a polynomial and is an integer.
- Divide by : Now, divide the second term by ., because , and .
- Divide second term: Finally, divide the constant term by . remains as it is because we can't simplify it further without knowing the value of .
- Divide constant term: Putting it all together, we get the polynomial part and the remainder.So, the answer is with a remainder of .
More problems from Find the inverse of a linear function
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help