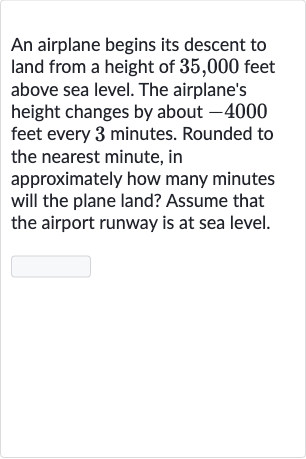
An airplane begins its descent to land from a height of 35,000 feet above sea level. The airplane's height changes by about -4000 feet every 3 minutes. Rounded to the nearest minute, in approximately how many minutes will the plane land? Assume that the airport runway is at sea level.

Question

Bytelearn · Accepted Answer

Calculate Total Descent Distance: Determine the total distance the airplane needs to descend to reach sea level from 35,000 feet. Since the airplane is at 35,000 feet and needs to reach sea level, which is 0 feet, it needs to descend a total of 35,000 feet.Calculate Rate of Descent: Calculate the rate of descent per minute. The airplane descends -4000 feet every 3 minutes. To find the descent per minute, divide -4000 feet by 3 minutes. -4000 feet / 3 minutes = -1333.33 feet per minute (rounded to two decimal places).Calculate Total Descent Time: Calculate the total time required for the airplane to descend 35,000 feet at the rate of -1333.33 feet per minute. To find the time, divide the total distance to descend by the rate of descent per minute. 35,000 feet / -1333.33 feet per minute = -26.25 minutes.Take Absolute Value of Time: Since we cannot have a negative time, we take the absolute value of the time calculated in Step 3. Absolute value of -26.25 minutes is 26.25 minutes.Round Time to Nearest Minute: Round the time to the nearest minute. 26.25 minutes rounded to the nearest minute is 26 minutes.

An airplane begins its descent to land from a height of $35$ , $000$ feet above sea level. The airplane's height changes by about $-4000$ feet every $3$ minutes. Rounded to the nearest minute, in approximately how many minutes will the plane land? Assume that the airport runway is at sea level.

Full solution

More problems from Rate of travel: word problems

Related topics