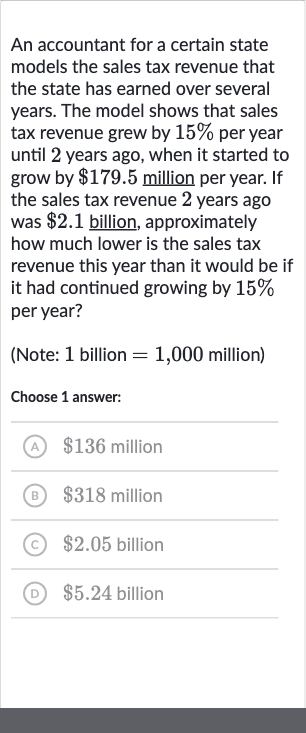
An accountant for a certain state models the sales tax revenue that the state has earned over several years. The model shows that sales tax revenue grew by 15% per year until 2 years ago, when it started to grow by $179.5 million per year. If the sales tax revenue 2 years ago was $2.1 billion, approximately how much lower is the sales tax revenue this year than it would be if it had continued growing by 15% per year? (Note: 1 billion =1,000 million) Choose 1 answer: (A) $136 million (B) $318 million (c) $2.05 billion (D) $5.24 billion

Question

An accountant for a certain state models the sales tax revenue that the state has earned over several years. The model shows that sales tax revenue grew by  15% per year until 2 years ago, when it started to grow by  $179.5 million per year. If the sales tax revenue 2 years ago was  $2.1 billion, approximately how much lower is the sales tax revenue this year than it would be if it had continued growing by  15% per year? (Note: 1 billion  =1,000 million) Choose 1 answer: (A)  $136 million (B)  $318 million (c)  $2.05 billion (D)  $5.24 billion

Bytelearn · Accepted Answer

Rephrase Question: First, let's rephrase the  ＂How much lower is the current year's sales tax revenue compared to what it would have been if it had continued to grow by 15% per year instead of by a flat $179.5 million per year, given that the revenue was $2.1 billion 2 years ago?＂Calculate 15% Growth: To find out the sales tax revenue if it had continued to grow by 15% per year, we need to calculate the revenue for the past two years with a 15% increase each year. Starting with $2.1 billion, we first calculate the revenue for the first year after the change. Revenue after 1 year at 15% growth = $2.1 billion * (1 + 0.15)Calculate 1st Year: Perform the calculation for the first year's revenue with a 15% increase. Revenue after 1 year at 15% growth = $2.1 billion * 1.15 Revenue after 1 year at 15% growth = $2.415 billionCalculate 2nd Year: Now, calculate the revenue for the second year with another 15% increase on the first year's revenue. Revenue after 2 years at 15% growth = $2.415 billion * (1 + 0.15)Calculate Flat Increase: Perform the calculation for the second year's revenue with a 15% increase. Revenue after 2 years at 15% growth = $2.415 billion * 1.15 Revenue after 2 years at 15% growth = $2.77725 billionCalculate Actual Revenue: Next, we calculate the actual revenue for the past two years with a flat increase of $179.5 million per year. Starting again with $2.1 billion, we add $179.5 million for each of the two years. Actual revenue after 1 year = $2.1 billion + $179.5 million Actual revenue after 2 years = ($2.1 billion + $179.5 million) + $179.5 millionFind the Difference: Perform the calculation for the actual revenue after two years. Actual revenue after 1 year = $2.1 billion + $0.1795 billion Actual revenue after 1 year = $2.2795 billion Actual revenue after 2 years = $2.2795 billion + $0.1795 billion Actual revenue after 2 years = $2.459 billionCalculate the Difference: Now, we find the difference between the projected revenue if it had grown by 15% per year and the actual revenue with the flat increase. Difference = Projected revenue after 2 years at 15% growth - Actual revenue after 2 years Difference = $2.77725 billion - $2.459 billionRound to Nearest Million: Perform the calculation for the difference. Difference = $2.77725 billion - $2.459 billion Difference = $0.31825 billion Difference = $318.25 millionSince we need to approximate the difference and the options are given in whole millions, we round the difference to the nearest million. Approximate difference = $318 million

Full solution

More problems from Find probabilities using combinations and permutations

Related topics