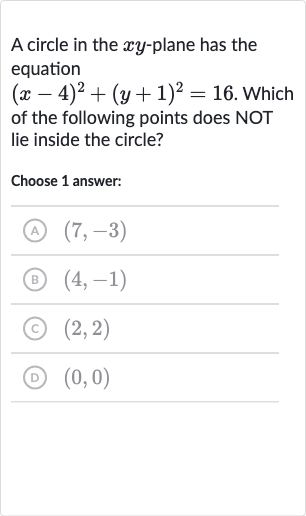
A circle in the xy-plane has the equation (x-4)^(2)+(y+1)^(2)=16. Which of the following points does NOT lie inside the circle? Choose 1 answer: (A) (7,-3) (B) (4,-1) (c) (2,2) (D) (0,0)

Question

A circle in the  xy-plane has the equation  (x-4)^(2)+(y+1)^(2)=16. Which of the following points does NOT lie inside the circle? Choose 1 answer: (A)  (7,-3) (B)  (4,-1) (c)  (2,2) (D)  (0,0)

Bytelearn · Accepted Answer

Equation of the Circle: The equation of the circle is given by (x-4)^(2)+(y+1)^(2)=16. The center of the circle is at (4, -1) and the radius is the square root of 16, which is 4. To determine if a point lies inside the circle, we can plug the coordinates of the point into the circle's equation and see if the resulting value is less than 16. If it is, the point lies inside the circle; if it is equal to 16, the point lies on the circle; and if it is greater than 16, the point lies outside the circle.Checking Point (A): Let's check point (A) (7, -3). Substitute x = 7 and y = -3 into the circle's equation: (7-4)^(2)+(-3+1)^(2) = (3)^(2)+(2)^(2) = 9 + 4 = 13. Since 13 is less than 16, point (A) lies inside the circle.Checking Point (B): Now, let's check point (B) (4, -1). Substitute x = 4 and y = -1 into the circle's equation: (4-4)^(2)+(-1+1)^(2) = (0)^(2)+(0)^(2) = 0 + 0 = 0. Since 0 is less than 16, point (B) lies inside the circle.Checking Point (C): Next, let's check point (C) (2, 2). Substitute x = 2 and y = 2 into the circle's equation: (2-4)^(2)+(2+1)^(2) = (-2)^(2)+(3)^(2) = 4 + 9 = 13. Since 13 is less than 16, point (C) lies inside the circle.Checking Point (D): Finally, let's check point (D) (0, 0). Substitute x = 0 and y = 0 into the circle's equation: (0-4)^(2)+(0+1)^(2) = (-4)^(2)+(1)^(2) = 16 + 1 = 17. Since 17 is greater than 16, point (D) does NOT lie inside the circle.

A circle in the $x y$ -plane has the equation $\newline$ $(x-4)^{2}+(y+1)^{2}=16$ . Which of the following points does NOT lie inside the circle? $\newline$ Choose $1$ answer: $\newline$ (A) $(7,-3)$ $\newline$ (B) $(4,-1)$ $\newline$ (C) $(2,2)$ $\newline$ (D) $(0,0)$

Full solution

More problems from Slopes of parallel and perpendicular lines

Related topics