AI tutor
Full solution
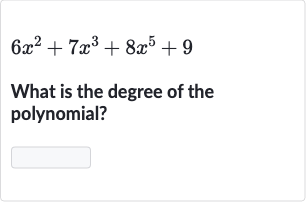
Q. What is the degree of the polynomial?
- Identifying the highest power: The degree of a polynomial is the highest power of the variable that appears in the polynomial with a non-zero coefficient. To find the degree, we need to identify the term with the highest exponent of .
- Analyzing the given polynomial: Looking at the given polynomial , we can see that the terms are , , , and . The term is a constant term and does not affect the degree of the polynomial.
- Determining the degree: The term with the highest exponent of x is . Therefore, the degree of the polynomial is .