Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

3y^(2)+5y-10=0
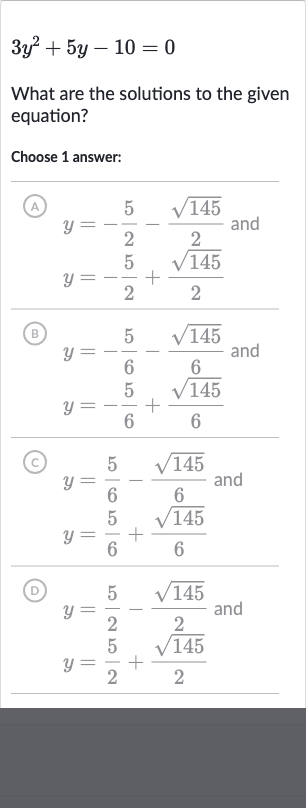
What are the solutions to the given equation?
Choose 1 answer:
(A)
y=-(5)/(2)-(sqrt145)/(2) and

y=-(5)/(2)+(sqrt145)/(2)
(B)
y=-(5)/(6)-(sqrt145)/(6) and

y=-(5)/(6)+(sqrt145)/(6)
(c)
y=(5)/(6)-(sqrt145)/(6) and

y=(5)/(6)+(sqrt145)/(6)
(D)
y=(5)/(2)-(sqrt145)/(2) and

y=(5)/(2)+(sqrt145)/(2)

$3 y^{2}+5 y-10=0$ $\newline$ What are the solutions to the given equation? $\newline$ Choose $1$ answer: $\newline$ (A) $y=-\frac{5}{2}-\frac{\sqrt{145}}{2}$ and $\newline$ $y=-\frac{5}{2}+\frac{\sqrt{145}}{2}$ $\newline$ (B) $y=-\frac{5}{6}-\frac{\sqrt{145}}{6}$ and $\newline$ $y=-\frac{5}{6}+\frac{\sqrt{145}}{6}$ $\newline$ (C) $y=\frac{5}{6}-\frac{\sqrt{145}}{6}$ and $\newline$ $y=\frac{5}{6}+\frac{\sqrt{145}}{6}$ $\newline$ (D) $y=\frac{5}{2}-\frac{\sqrt{145}}{2}$ and $\newline$ $y=\frac{5}{2}+\frac{\sqrt{145}}{2}$

View step-by-step help

View step-by-step help

Compare linear and exponential growth

Full solution

Q.

3 y^{2}+5 y-10=0

\newline

What are the solutions to the given equation?

\newline

Choose

1

answer:

\newline

(A)

y=-\frac{5}{2}-\frac{\sqrt{145}}{2}

and

\newline

y=-\frac{5}{2}+\frac{\sqrt{145}}{2}

\newline

(B)

y=-\frac{5}{6}-\frac{\sqrt{145}}{6}

and

\newline

y=-\frac{5}{6}+\frac{\sqrt{145}}{6}

\newline

(C)

y=\frac{5}{6}-\frac{\sqrt{145}}{6}

and

\newline

y=\frac{5}{6}+\frac{\sqrt{145}}{6}

\newline

(D)

y=\frac{5}{2}-\frac{\sqrt{145}}{2}

and

\newline

y=\frac{5}{2}+\frac{\sqrt{145}}{2}

Identify equation type and method: Identify the type of equation and the method to solve it. $\newline$ The given equation is a quadratic equation in the form $ay^2 + by + c = 0$ , where $a = 3$ , $b = 5$ , and $c = -10$ . To solve this equation, we can use the quadratic formula $y = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}$ .
Apply quadratic formula: Apply the quadratic formula to find the solutions for $y$ . $\newline$ Using the quadratic formula, we have: $\newline$ $y = \frac{{-\left(5\right) \pm \sqrt{{\left(5\right)^2 - 4\left(3\right)\left(-10\right)}}}}{{2\left(3\right)}}$ $\newline$ $y = \frac{{-5 \pm \sqrt{25 + 120}}}{{6}}$ $\newline$ $y = \frac{{-5 \pm \sqrt{145}}}{{6}}$
Simplify solutions: Simplify the solutions. $\newline$ We have two possible solutions based on the extpm{} sign in the quadratic formula: $\newline$ y = ( $-5$ + \sqrt{ $145$ }) / $6$ and y = ( $-5$ - \sqrt{ $145$ }) / $6$
Check solutions against options: Check the solutions against the given options. $\newline$ The solutions we found match with option (B): $\newline$ y = $-\frac{5}{6} - \frac{\sqrt{145}}{6}$ and y = $-\frac{5}{6} + \frac{\sqrt{145}}{6}$

More problems from Compare linear and exponential growth

Question

We want to factor the following expression:

\newline

x^{4}+10 x+25

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

(x-2)^{4}-(y+3)^{4}

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

(x-1)^{2}+(x-1)+4

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

9 x^{4}+4 y^{2}

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

16 x^{4}-25

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

x^{4}+3 x^{2} y+9 y^{2}

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

(x+5)^{4}+2(x+5)^{2}-1

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

(x-3)^{2}-10(x-3)+25

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

(x+7)^{2}+2 y(x+7)+y^{2}

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

9 x^{6}+6 x^{3} y+y^{2}

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant