Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

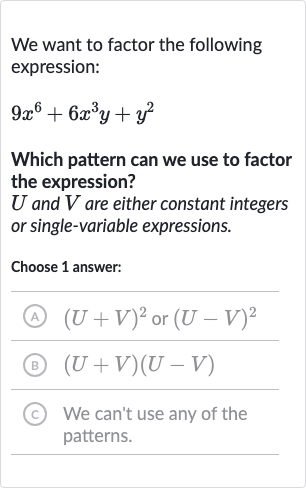
We want to factor the following expression:

9x^(6)+6x^(3)y+y^(2)
Which pattern can we use to factor the expression?

U and
V are either constant integers or single-variable expressions.
Choose 1 answer:
(A)
(U+V)^(2) or
(U-V)^(2)
(B)
(U+V)(U-V)
(c) We can't use any of the patterns.

We want to factor the following expression: $\newline$ $9 x^{6}+6 x^{3} y+y^{2}$ $\newline$ Which pattern can we use to factor the expression? $\newline$ $U$ and $V$ are either constant integers or single-variable expressions. $\newline$ Choose $1$ answer: $\newline$ (A) $(U+V)^{2}$ or $(U-V)^{2}$ $\newline$ (B) $(U+V)(U-V)$ $\newline$ (C) We can't use any of the patterns.

View step-by-step help

View step-by-step help

Compare linear and exponential growth

Full solution

Q. We want to factor the following expression:

\newline

9 x^{6}+6 x^{3} y+y^{2}

\newline

Which pattern can we use to factor the expression?

\newline

U

and

V

are either constant integers or single-variable expressions.

\newline

Choose

1

answer:

\newline

(A)

(U+V)^{2}

or

(U-V)^{2}

\newline

(B)

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Recognize the structure: Recognize the structure of the given expression. $\newline$ The given expression is $9x^{6} + 6x^{3}y + y^{2}$ . We can observe that the first term is a perfect square $(3x^{3})^2$ , the last term is a perfect square $(y)^2$ , and the middle term is twice the product of the square roots of the first and last terms $(2 \times 3x^{3} \times y)$ .
Identify the factoring pattern: Identify the factoring pattern. $\newline$ The structure of the expression matches the pattern of a perfect square trinomial, which is $(A + B)^2 = A^2 + 2AB + B^2$ or $(A - B)^2 = A^2 - 2AB + B^2$ . In this case, since the middle term is positive, we will use the $(A + B)^2$ pattern.
Apply the factoring pattern: Apply the factoring pattern to the expression. $\newline$ We can write the expression as $(3x^{3} + y)^2$ because $(3x^{3})^2 = 9x^{6}$ , $2 \cdot 3x^{3} \cdot y = 6x^{3}y$ , and $y^2 = y^{2}$ . Therefore, the factored form of the expression is $(3x^{3} + y)^2$ .

More problems from Compare linear and exponential growth

Question

We want to factor the following expression:

\newline

x^{4}+10 x+25

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

(x-2)^{4}-(y+3)^{4}

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

(x-1)^{2}+(x-1)+4

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

9 x^{4}+4 y^{2}

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

16 x^{4}-25

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

x^{4}+3 x^{2} y+9 y^{2}

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

(x+5)^{4}+2(x+5)^{2}-1

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

(x-3)^{2}-10(x-3)+25

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

We want to factor the following expression:

\newline

(x+7)^{2}+2 y(x+7)+y^{2}

\newline

Which pattern can we use to factor the expression?

\newline

U

V

are either constant integers or single-variable expressions.

\newline

1

\newline

(U+V)^{2}

(U-V)^{2}

\newline

(U+V)(U-V)

\newline

(C) We can't use any of the patterns.

Posted 8 months ago

Question

20\%

more goblins than wizards in magic club. There are

120

goblins in magic club. How many wizards are in the magic club? wizards

Posted 2 hours ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant