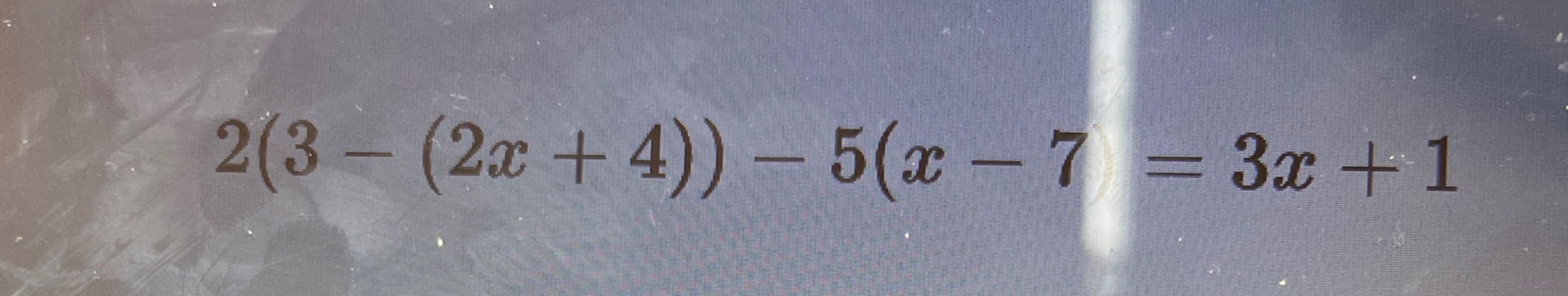AI tutor
Full solution
Q. Solve for .
- Distribute into parentheses: Distribute the into the parentheses .
- Combine like terms: Combine like terms from the result of Step .
- Distribute into parentheses: Distribute the into the parentheses .
- Combine results: Combine the results from Step and Step .
- Combine like terms: Combine like terms from the result of Step .So,
- Write original equation: Write down the original equation with the simplified left side from Step .
- Move terms containing : Move all terms containing to one side of the equation.Add to both sides.
- Combine like terms: Combine like terms and isolate the variable . Subtract from both sides.
- Divide both sides: Divide both sides by to solve for .