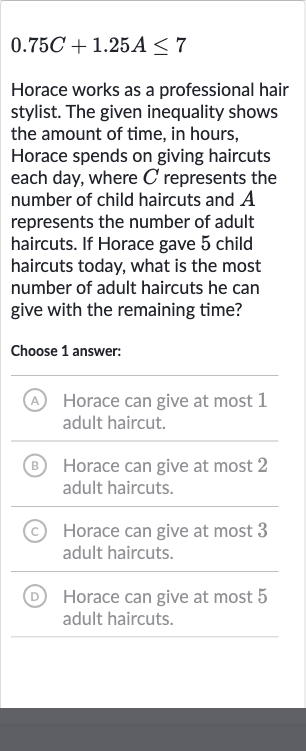
$0.75 C+1.25 A \leq 7$ $\newline$ Horace works as a professional hair stylist. The given inequality shows the amount of time, in hours, Horace spends on giving haircuts each day, where $C$ represents the number of child haircuts and $A$ represents the number of adult haircuts. If Horace gave $5$ child haircuts today, what is the most number of adult haircuts he can give with the remaining time? $\newline$ Choose $1$ answer: $\newline$ (A) Horace can give at most $1$ adult haircut. $\newline$ (B) Horace can give at most $2$ adult haircuts. $\newline$ (C) Horace can give at most $3$ adult haircuts. $\newline$ (D) Horace can give at most $5$ adult haircuts.

View step-by-step help

Home

Math Problems

Algebra 2

Solve polynomial equations

Full solution

0.75 C+1.25 A \leq 7

\newline

Horace works as a professional hair stylist. The given inequality shows the amount of time, in hours, Horace spends on giving haircuts each day, where

C

represents the number of child haircuts and

A

represents the number of adult haircuts. If Horace gave

5

child haircuts today, what is the most number of adult haircuts he can give with the remaining time?

\newline

Choose

1

answer:

\newline

(A) Horace can give at most

1

adult haircut.

\newline

(B) Horace can give at most

2

adult haircuts.

\newline

3

adult haircuts.

\newline

(D) Horace can give at most

5

adult haircuts.

Substitute and solve: Substitute the number of child haircuts $C = 5$ into the inequality to find the time remaining for adult haircuts. $0.75C + 1.25A \leq 7$ $0.75(5) + 1.25A \leq 7$ $3.75 + 1.25A \leq 7$
Isolate the term with A: Subtract $3.75$ from both sides to isolate the term with A. $\newline$ $1.25A \leq 7 - 3.75$ $\newline$ $1.25A \leq 3.25$
Solve for A: Divide both sides by $1.25$ to solve for $A$ . $\newline$ $A \leq \frac{3.25}{1.25}$ $\newline$ $A \leq 2.6$
Maximum number of adult haircuts: Since $A$ represents the number of adult haircuts and must be a whole number, Horace can give at most $2$ adult haircuts because he cannot give a fraction of a haircut.