Full solution
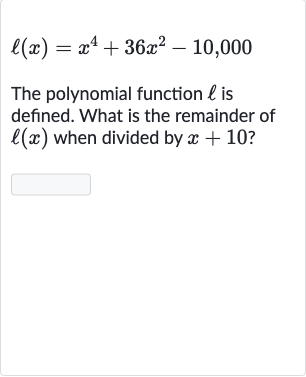
Q. The polynomial function is defined. What is the remainder of when divided by ?
- Use Remainder Theorem: To find the remainder of when divided by , we can use the Remainder Theorem. The Remainder Theorem states that the remainder of a polynomial divided by is . Since we are dividing by , we will find .
- Substitute : Substitute into the polynomial .
- Calculate value of : Calculate the value of .
- Simplify expression: Simplify the expression to find the remainder.
More problems from Composition of linear and quadratic functions: find a value
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help