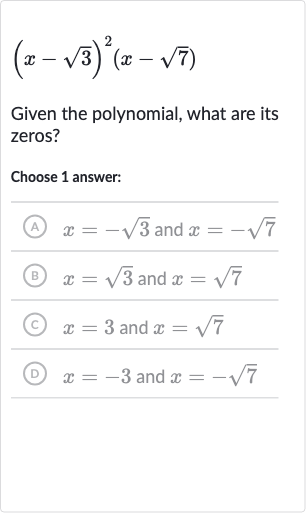
Full solution
Q. Given the polynomial, what are its zeros?Choose answer:(A) and (B) and (C) and (D) and
- Factored Polynomial: Factored polynomial: Which equation finds the zeros for this polynomial?Set the polynomial equal to zero.
- Finding Zeros: First, consider the factor .What is the value of ?Take the square root of both sides.
- Solving : Now, consider the factor .What is the value of ?
- Solving : We have found the zeros of the polynomial:These are the roots of the polynomial.