Write a linear equation from a slope and y-intercept

Full solution

-12 x^{2}-t x+t=0

\newline

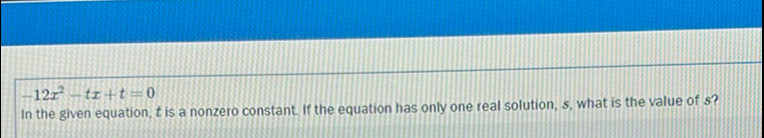
In the given equation,

t

is a nonzero constant. If the equation has only one real solution,

s

, what is the value of

s

Understand Conditions for Real Solution: To find the value of the real solution $s$ , we need to understand the conditions for a quadratic equation to have only one real solution. A quadratic equation $ax^2 + bx + c = 0$ has only one real solution when its discriminant is zero. The discriminant is given by the formula $b^2 - 4ac$ .
Calculate Discriminant: In the given equation $-12x^2 - tx + t = 0$ , we can identify $a = -12$ , $b = -t$ , and $c = t$ . Let's calculate the discriminant using these values. $\newline$ Discriminant ( $D$ ) = $b^2 - 4ac = (-t)^2 - 4(-12)(t) = t^2 + 48t$ .
Set Discriminant Equal to Zero: For the equation to have only one real solution, the discriminant must be zero. Therefore, we set the discriminant equal to zero and solve for $t$ . $t^2 + 48t = 0$
Factorize and Solve for $t$ : We can factor out $t$ from the equation: $t(t + 48) = 0$
Find Value of $t$ : Since $t$ is a nonzero constant, we cannot have $t = 0$ . Therefore, the only solution for $t$ is when $t + 48 = 0$ , which gives us $t = -48$ .
Calculate Vertex for Real Solution: Now that we have the value of $t$ , we can find the value of the real solution $s$ . Since the equation has only one real solution, this solution is also the vertex of the parabola represented by the quadratic equation. The $x$ -coordinate of the vertex is given by $-\frac{b}{2a}$ .
Calculate Vertex for Real Solution: Now that we have the value of $t$ , we can find the value of the real solution $s$ . Since the equation has only one real solution, this solution is also the vertex of the parabola represented by the quadratic equation. The $x$ -coordinate of the vertex is given by $-\frac{b}{2a}$ .Substitute $a = -12$ and $b = -t$ (with $t = -48$ ) into the vertex formula to find $s$ : $s = -\frac{-t}{2a} = -\frac{-(-48)}{2(-12)} = \frac{48}{-24} = -2.$