AI tutor
Full solution
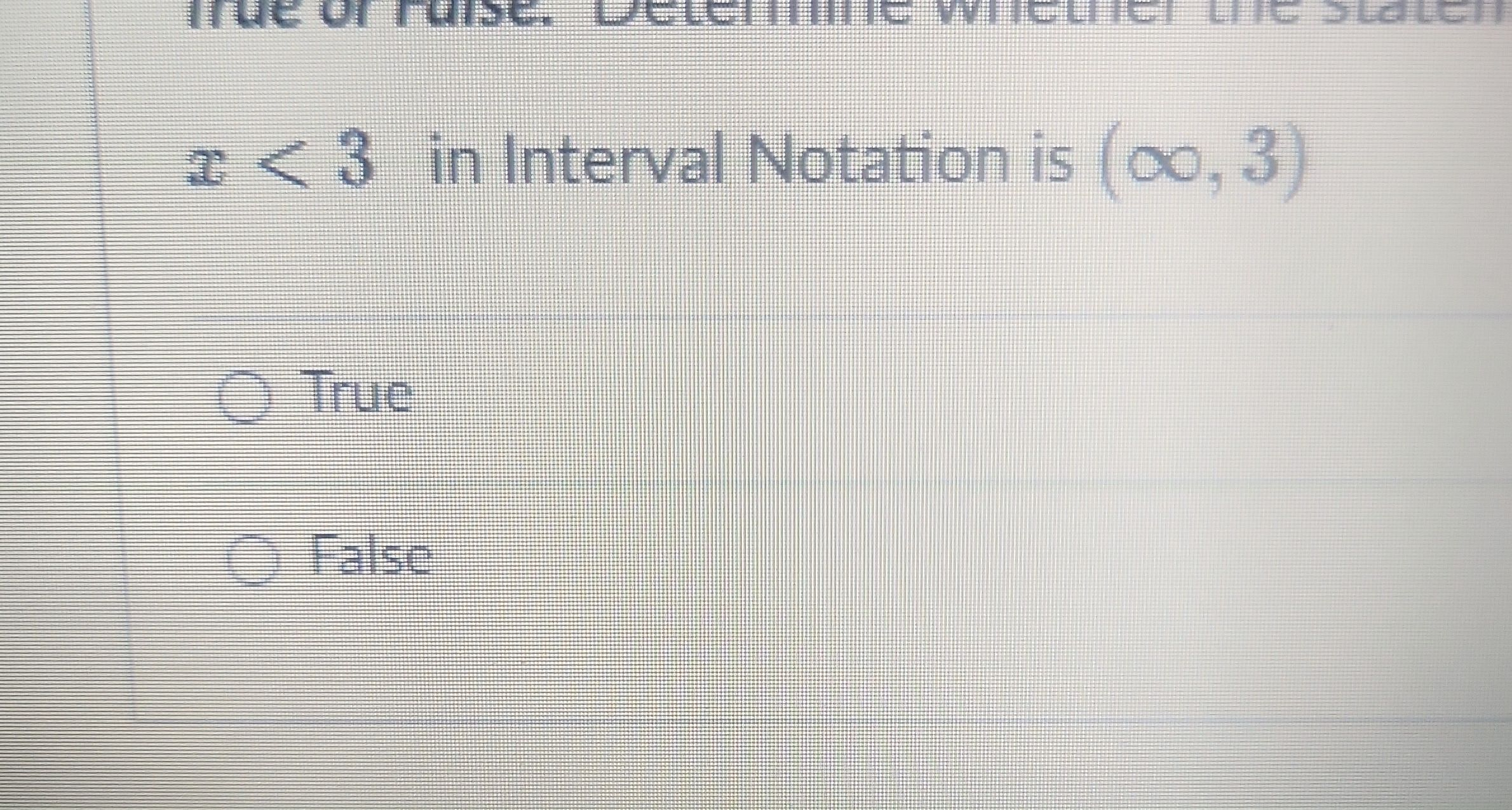
Q. in Interval Notation is TrueFalse
- Identify Values Less Than : To represent the inequality x < 3 in interval notation, we need to consider all the values of that are less than . The interval notation for this inequality should start from negative infinity, since there is no lower bound to the values can take, as long as they are less than . The interval should not include itself, since is strictly less than , not less than or equal to . Therefore, the correct interval notation is written with a parenthesis to indicate that is not included in the interval.
- Interval Notation Explanation: The correct interval notation for x < 3 is written as , where the parenthesis around indicates that is not included in the interval. The notation is attempting to represent negative infinity by "oo," which is a common informal representation of infinity. However, in formal mathematical notation, infinity is represented by the symbol "".
- Comparison of Notations: Comparing the given interval notation with the correct formal notation \-\infty, 3, we can see that the only difference is the representation of infinity. The statement is true if we interpret "\infty" as the informal representation of negative infinity. However, in formal mathematics, we should use the symbol "\infty" to represent infinity.
More problems from Domain and range of quadratic functions: equations
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help