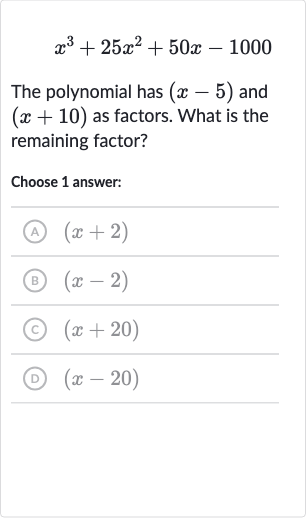
x^(3)+25x^(2)+50 x-1000 The polynomial has (x-5) and (x+10) as factors. What is the remaining factor? Choose 1 answer: (A) (x+2) (B) (x-2) (c) (x+20) (D) (x-20)

Question

x^(3)+25x^(2)+50 x-1000 The polynomial has  (x-5) and  (x+10) as factors. What is the remaining factor? Choose 1 answer: (A)  (x+2) (B)  (x-2) (c)  (x+20) (D)  (x-20)

Bytelearn · Accepted Answer

Step 1: Divide by (x-5): Given that (x-5) and (x+10) are factors of the polynomial x^3 + 25x^2 + 50x - 1000, we can start by dividing the polynomial by one of these factors to simplify it. Let's start by dividing the polynomial by (x-5).Step 2: Polynomial Division: Perform the polynomial division of x^3 + 25x^2 + 50x - 1000 by (x-5).Step 3: Quadratic Polynomial Result: The division process should yield a quadratic polynomial as the result. This quadratic polynomial, when multiplied by (x-5), should give us the original polynomial x^3 + 25x^2 + 50x - 1000.Step 4: Divide by (x+10): After dividing by (x-5), we find that the resulting quadratic polynomial is x^2 + 30x + 200. This is because (x-5)(x^2 + 30x + 200) = x^3 + 25x^2 + 50x - 1000.Step 5: Polynomial Division: Now, we need to divide the quadratic polynomial x^2 + 30x + 200 by the other given factor, (x+10), to find the remaining factor.Step 6: Linear Polynomial Result: Perform the polynomial division of x^2 + 30x + 200 by (x+10).Step 7: Remaining Factor: The division process should yield a linear polynomial as the result. This linear polynomial, when multiplied by (x+10), should give us the quadratic polynomial x^2 + 30x + 200.After dividing by (x+10), we find that the resulting linear polynomial is x + 20. This is because (x+10)(x + 20) = x^2 + 30x + 200.Therefore, the remaining factor of the polynomial x^3 + 25x^2 + 50x - 1000, given that (x-5) and (x+10) are factors, is (x + 20).

$x^{3}+25 x^{2}+50 x-1000$ $\newline$ The polynomial has $(x-5)$ and $(x+10)$ as factors. What is the remaining factor? $\newline$ Choose $1$ answer: $\newline$ (A) $(x+2)$ $\newline$ (B) $(x-2)$ $\newline$ (C) $(x+20)$ $\newline$ (D) $(x-20)$

Full solution

More problems from Factor sums and differences of cubes

Related topics