Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

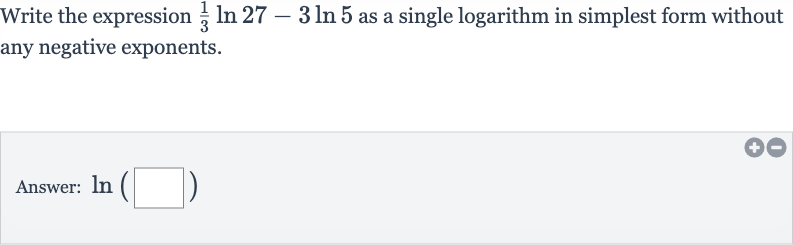
Write the expression
(1)/(3)ln 27-3ln 5 as a single logarithm in simplest form without any negative exponents.
Answer:
ln(◻)

Write the expression $\frac{1}{3} \ln 27-3 \ln 5$ as a single logarithm in simplest form without any negative exponents. $\newline$ Answer: $\ln (\square)$

View step-by-step help

View step-by-step help

Power rule with rational exponents

Full solution

Q. Write the expression

\frac{1}{3} \ln 27-3 \ln 5

as a single logarithm in simplest form without any negative exponents.

\newline

Answer:

\ln (\square)

Apply power rule: We have the expression $(\frac{1}{3})\ln 27 - 3\ln 5$ . We will use the logarithm power rule, which states that $a \cdot \ln(b) = \ln(b^a)$ , to simplify each term.
Calculate cube root: Apply the power rule to the first term: $(\frac{1}{3})\ln 27 = \ln(27^{\frac{1}{3}})$ . $\newline$ Calculate $27^{\frac{1}{3}}$ , which is the cube root of $27$ . $\newline$ $27^{\frac{1}{3}} = 3$ because $3^3 = 27$ . $\newline$ So, $(\frac{1}{3})\ln 27 = \ln(3)$ .
Apply power rule: Apply the power rule to the second term: $3\ln 5 = \ln(5^3)$ . Calculate $5^3$ . $5^3 = 125$ . So, $3\ln 5 = \ln(125)$ .
Combine logarithms: Now we have $\ln(3) - \ln(125)$ . We will use the logarithm quotient rule, which states that $\ln(a) - \ln(b) = \ln\left(\frac{a}{b}\right)$ , to combine the two logarithms into one.
Simplify to $\ln(\frac{3}{125})$ : Combine the logarithms: $\ln(3) - \ln(125) = \ln(\frac{3}{125})$ .
Simplify to $\ln(\frac{3}{125})$ : Combine the logarithms: $\ln(3) - \ln(125) = \ln(\frac{3}{125})$ .The expression is now simplified to a single logarithm: $\ln(\frac{3}{125})$ .

More problems from Power rule with rational exponents

Question

\newline

81^{\frac{1}{2}}

\newline

Posted 9 months ago

Question

\newline

(-32)^{1/5}

\newline

Posted 9 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{w^{\frac{4}{3}}}{w^{\frac{7}{3}}}

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 5 months ago

Question

Simplify. Assume all variables are positive.

\newline

(2r^{\frac{1}{3}})^8

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 5 months ago

Question

Simplify. Assume all variables are positive.

\newline

v^{\frac{7}{4}} \cdot v^{\frac{9}{4}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 5 months ago

Question

\newline

1^{\frac{1}{4}} \cdot 1^{\frac{1}{4}}

\newline

Posted 5 months ago

Question

\newline

32^{(-1/5)}

Posted 7 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{w^{\frac{3}{2}}}{w^{\frac{5}{2}}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 9 months ago

Question

Simplify. Assume all variables are positive.

\newline

(27x)^{\frac{2}{3}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 8 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{x^{\frac{1}{4}}}{x^{\frac{11}{4}}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 7 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant