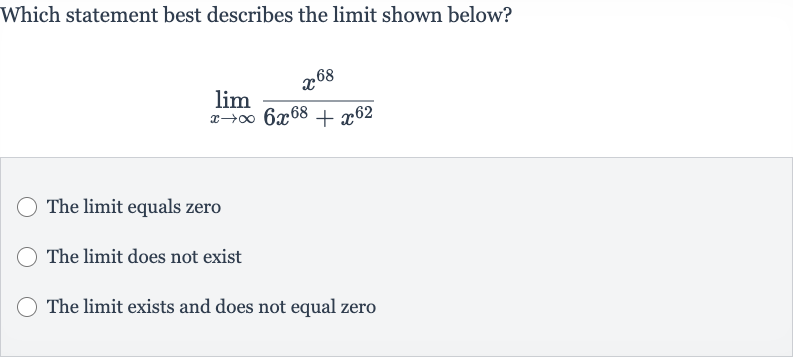Full solution
Q. Which statement best describes the limit shown below?The limit equals zeroThe limit does not existThe limit exists and does not equal zero
- Analyze Degree Comparison: We are given the limit expression . To find the limit as approaches infinity, we can compare the degrees of the polynomials in the numerator and the denominator.
- Divide by Highest Degree Term: Since the highest degree term in both the numerator and the denominator is , we can divide every term by to simplify the expression.
- Simplify Expression: Simplify the expression inside the limit by canceling out from the denominator's second term.
- Negligible Term Removal: As approaches infinity, approaches . Therefore, the term in the denominator becomes negligible.
- Final Simplification: Simplify the expression by removing the term that approaches zero.
- Limit Result: The limit of the function as approaches infinity is , which is a finite number and does not equal zero.
More problems from Power rule
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help