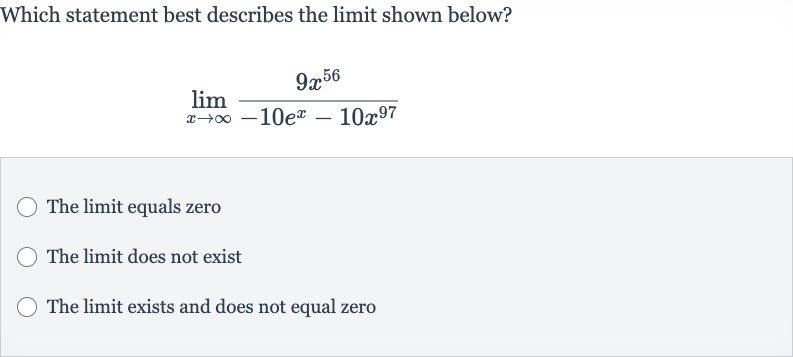Full solution
Q. Which statement best describes the limit shown below?The limit equals zeroThe limit does not existThe limit exists and does not equal zero
- Analyze Behavior as Approaches Infinity: We need to analyze the behavior of the function as approaches infinity. To do this, we look at the degrees of the polynomial in the numerator and the terms in the denominator.
- Degree of Numerator and Denominator: The numerator is , which is a polynomial of degree . The denominator is , which contains an exponential function and a polynomial of degree .
- Exponential Function Dominance: As approaches infinity, the exponential function grows much faster than any polynomial. Therefore, the term in the denominator will dominate over the term.
- Denominator Grows Faster: Since the exponential function grows faster than any power of , the denominator will grow much faster than the numerator as approaches infinity. This means that the fraction as a whole will approach zero.
- Limit as x Approaches Infinity: Therefore, the limit of as x approaches infinity is .
More problems from Power rule
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help