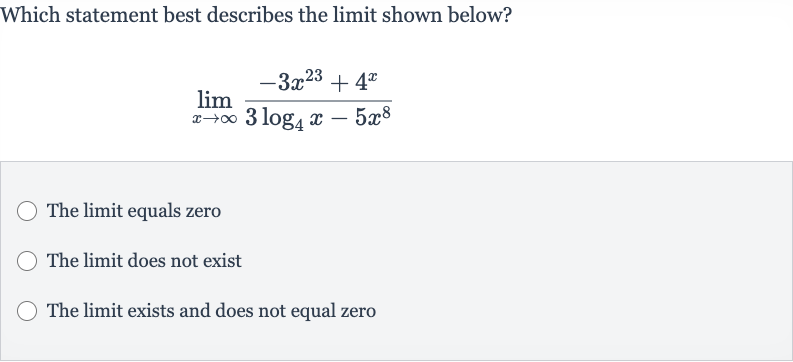Which statement best describes the limit shown below? lim_(x rarr oo)(-3x^(23)+4^(x))/(3log_(4)x-5x^(8)) The limit equals zero The limit does not exist The limit exists and does not equal zero

Question

Which statement best describes the limit shown below?  lim_(x rarr oo)(-3x^(23)+4^(x))/(3log_(4)x-5x^(8)) The limit equals zero The limit does not exist The limit exists and does not equal zero

Bytelearn · Accepted Answer

Given Limit Expression: We are given the limit expression: lim_(x → ∞)(-3x^(23) + 4^(x)) / (3log_(4)x - 5x^(8))  To find the behavior of this function as x approaches infinity, we need to determine the dominant terms in the numerator and the denominator.Determining Dominant Terms: In the numerator, the dominant term is -3x^(23) because as x approaches infinity, x^(23) will grow much faster than 4^(x), which is exponential but does not depend on x in the base.  In the denominator, the dominant term is -5x^(8) because as x approaches infinity, x^(8) will grow much faster than the logarithmic term 3log_(4)x.  So, we can simplify the limit to focus on the dominant terms in the numerator and the denominator: lim_(x → ∞)(-3x^(23)) / (-5x^(8))Simplifying the Limit: Now, we simplify the expression by dividing the coefficients and subtracting the exponents of x: (-3/-5) * x^(23-8) = (3/5) * x^(15)  As x approaches infinity, x^(15) will also approach infinity. Therefore, the limit of the simplified expression as x approaches infinity is also infinity.Final Limit Evaluation: Since the limit of the simplified expression is infinity, the original limit also approaches infinity. This means that the limit does not equal zero and it does exist, but it does not equal a finite number.  Therefore, the correct statement is: The limit exists and does not equal zero.

Which statement best describes the limit shown below? $\newline$ $\lim _{x \rightarrow \infty} \frac{-3 x^{23}+4^{x}}{3 \log _{4} x-5 x^{8}}$ $\newline$ The limit equals zero $\newline$ The limit does not exist $\newline$ The limit exists and does not equal zero

Full solution

More problems from Power rule

Related topics