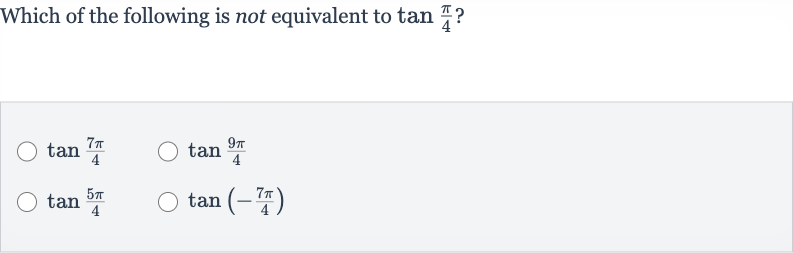AI tutor
Full solution
Q. Which of the following is not equivalent to ?
- Periodicity of Tangent Function: Understand the periodicity of the tangent function. The tangent function has a period of , which means that for any integer .
- Evaluate : Evaluate .The value of is because the tangent of an angle in a right triangle with equal sides is .
- Evaluate : Evaluate . Using the periodicity of the tangent function, is equivalent to which is . Since , = = .
- Evaluate : Evaluate . Using the periodicity of the tangent function, is equivalent to which is . Therefore, = = .
- Evaluate : Evaluate . Using the periodicity of the tangent function, is equivalent to which is . Therefore, .
- Evaluate : Evaluate . Using the periodicity of the tangent function, is equivalent to which is . Therefore, .
- Determine Non-Equivalent Option: Determine which option is not equivalent to . From the previous steps, we have determined that is the only option that is not equivalent to because it equals , while all the other options equal .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help