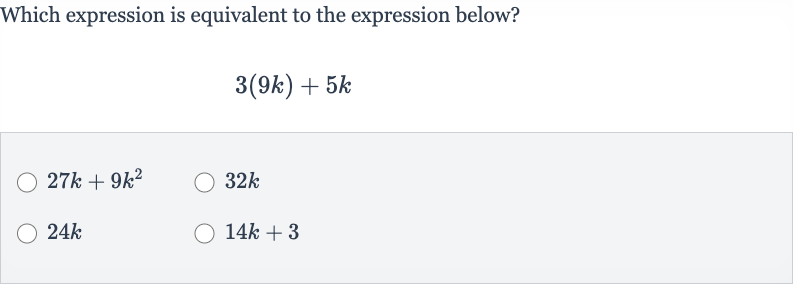Full solution
Q. Which expression is equivalent to the expression below?
- Distribute : Distribute the across the terms inside the parentheses in the expression .
Calculation:
So, becomes . - Combine like terms: Combine like terms in .Calculation:
- Compare with options: Compare the result with the given options.The expression matches one of the given options, which is "".
More problems from Simplify variable expressions involving like terms and the distributive property
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help