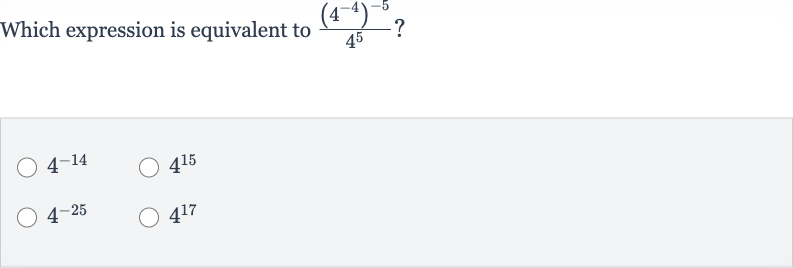AI tutor
Full solution
Q. Which expression is equivalent to
- Understand and Apply Power Rule: Understand the problem and apply the power of a power rule.We have the expression . According to the power of a power rule, . We will apply this rule to the numerator.
- Apply Power of Power Rule: Apply the power of a power rule to the numerator..
- Rewrite with Simplified Numerator: Rewrite the expression with the simplified numerator.Now the expression is .
- Apply Quotient of Powers Rule: Apply the quotient of powers rule.According to the quotient of powers rule, . We will apply this rule to the expression.
- Subtract Exponents: Subtract the exponents.
- Check Answer Choices: Check the answer choices to see which one matches our result.The expression matches one of the answer choices, which is .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help