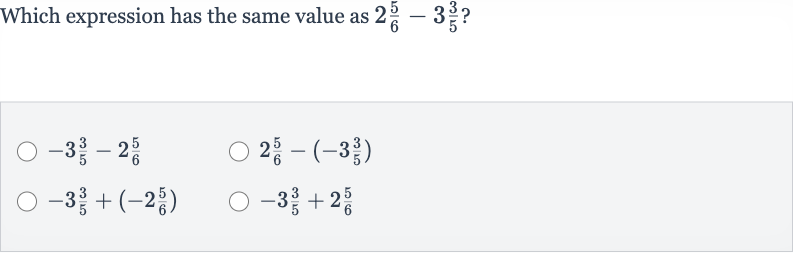Which expression has the same value as 2(5)/(6)-3(3)/(5) ? -3(3)/(5)-2(5)/(6) 2(5)/(6)-(-3(3)/(5)) -3(3)/(5)+(-2(5)/(6)) -3(3)/(5)+2(5)/(6)

Question

Which expression has the same value as  2(5)/(6)-3(3)/(5) ?  -3(3)/(5)-2(5)/(6)  2(5)/(6)-(-3(3)/(5))  -3(3)/(5)+(-2(5)/(6))  -3(3)/(5)+2(5)/(6)

Bytelearn · Accepted Answer

Understand the original expression: Understand the original expression. The original expression is 2(5)/(6)-3(3)/(5). We need to find which of the given options has the same value as this expression.Analyze the first option: Analyze the first option. The first option is -3(3)/(5)-2(5)/(6). This is the original expression with the terms reversed and without changing their signs. Reversing the order of subtraction will not yield the same result because subtraction is not commutative.Analyze the second option: Analyze the second option. The second option is 2(5)/(6)-(-3(3)/(5)). This is the original expression with the second term negated. This is equivalent to adding the absolute value of the second term to the first term, which is not the same as the original expression.Analyze the third option: Analyze the third option. The third option is -3(3)/(5)+(-2(5)/(6)). This option negates the second term of the original expression and adds it to the first term. This changes the operation from subtraction to addition, which does not yield the same result.Analyze the fourth option: Analyze the fourth option. The fourth option is -3(3)/(5)+2(5)/(6). This option keeps the first term of the original expression the same and changes the sign of the second term from negative to positive, effectively subtracting the second term from the first. This is the same as the original expression because subtracting a negative is the same as adding a positive.

Which expression has the same value as $2 \frac{5}{6}-3 \frac{3}{5}$ ? $\newline$ $-3 \frac{3}{5}-2 \frac{5}{6}$ $\newline$ $2 \frac{5}{6}-\left(-3 \frac{3}{5}\right)$ $\newline$ $-3 \frac{3}{5}+\left(-2 \frac{5}{6}\right)$ $\newline$ $-3 \frac{3}{5}+2 \frac{5}{6}$

Full solution

More problems from Multiplication with rational exponents

Related topics