Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

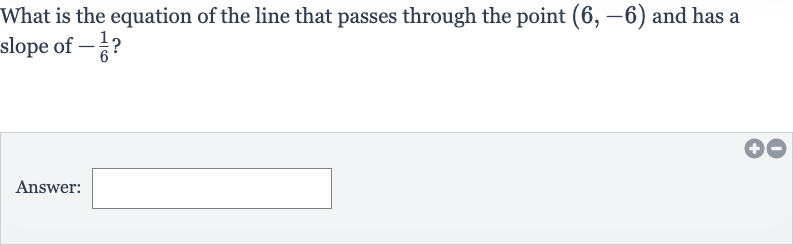
What is the equation of the line that passes through the point (6,-6) and has a slope of -(1)/(6) ? Answer:

What is the equation of the line that passes through the point (6,−6) (6,-6) and has a slope of −16 -\frac{1}{6} ?\newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Write the equation of a linear functionright chevron icon

Full solution

Q. What is the equation of the line that passes through the point (6,−6) (6,-6) and has a slope of −16 -\frac{1}{6} ?\newlineAnswer:
  1. Identify Slope and Point: Identify the slope mm and the point (x1,y1)(x_1, y_1) through which the line passes.\newlineThe slope mm is given as −16-\frac{1}{6}, and the point (x1,y1)(x_1, y_1) is (6,−6)(6, -6).
  2. Use Point-Slope Form: Use the point-slope form of the equation of a line to plug in the values of the slope and the point.\newlineThe point-slope form is y−y1=m(x−x1)y - y_1 = m(x - x_1).\newlineSubstitute m=−16m = -\frac{1}{6}, x1=6x_1 = 6, and y1=−6y_1 = -6 into the equation to get y−(−6)=−16(x−6)y - (-6) = -\frac{1}{6}(x - 6).
  3. Simplify Equation: Simplify the equation from the point-slope form to the slope-intercept form y=mx+by = mx + b. Start by distributing the slope on the right side of the equation: y+6=−16x+16⋅6y + 6 = -\frac{1}{6}x + \frac{1}{6}\cdot6.
  4. Isolate y: Continue simplifying the equation by performing the multiplication on the right side.\newlineThe equation becomes y+6=−16x+1y + 6 = -\frac{1}{6}x + 1.
  5. Combine Like Terms: Isolate yy to get the equation in slope-intercept form.\newlineSubtract 66 from both sides of the equation to get y=−16x+1−6y = -\frac{1}{6}x + 1 - 6.
  6. Combine Like Terms: Isolate yy to get the equation in slope-intercept form.\newlineSubtract 66 from both sides of the equation to get y=−16x+1−6y = -\frac{1}{6}x + 1 - 6.Combine like terms on the right side of the equation to find the y-intercept (b)(b).\newlineThe equation simplifies to y=−16x−5y = -\frac{1}{6}x - 5.

More problems from Write the equation of a linear function

Question
What is the domain of this function?\newline(9,–2)(6,–10)(–3,9)(5,2)(9,–2)(6,–10)(–3,9)(5,2)\newlineChoices:\newline(A){–3,5,6,9}(B){–10,–2,2,9}(C){2,5,6,9}(D){–6,–3,5,9}(A)\{–3,5,6,9\}\newline(B)\{–10,–2,2,9\}\newline(C)\{2,5,6,9\}\newline(D)\{–6,–3,5,9\}
Get tutor helpright-arrow

Posted 2 months ago

Question
Look at this set of ordered pairs:\newline(2,14)(2, 14)\newline(10,12)(10, 12)\newline(3,−19)(3, -19)\newlineIs this relation a function?\newlineChoices:\newline[[yes]\text{[[yes]}[no]]\text{[no]]}
Get tutor helpright-arrow

Posted 2 months ago

Question
Use the following function rule to find f(11) f(11) .\newlinef(x)=−10−7x f(x) = -10 - 7x \newlinef(11)= f(11) = _____
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the slope of the line y=−3x−25 y = -3x - \frac{2}{5} .\newlineSimplify your answer and write it as a proper fraction, improper fraction, or integer.\newline_____\_\_\_\_\_
Get tutor helpright-arrow

Posted 2 months ago

Question
A line has a slope of 33 and passes through the point (−2,−10)(-2,-10). Write its equation in slope-intercept form.\newlineWrite your answer using integers, proper fractions, and improper fractions in simplest form.
Get tutor helpright-arrow

Posted 2 months ago

Question
What is the range of this function?\newliney = |x|\newlineChoices:\newlineall real numbers\text{all real numbers}\newline{y∣y≥0}\{y \mid y \geq 0\}\newline{y∣y≤0}\{y \mid y \leq 0\}\newline{y∣y>0}\{y \mid y > 0\}
Get tutor helpright-arrow

Posted 3 months ago

Question
Find g(x) g(x) , where g(x) g(x) is the translation 1 1 unit down of f(x)=∣x∣ f(x) = |x| .\newlineWrite your answer in the form a∣x−h∣+k a|x - h| + k , where a a , h h , and k k are integers.\newlineg(x)= g(x) = ______\newline
Get tutor helpright-arrow

Posted 3 months ago

Question
What is the domain of this function?\newline(8,–1)(7,–11)(–4,10)(4,3)(8,–1)(7,–11)(–4,10)(4,3)\newlineChoices: \newline(A){–4,4,7,8}(A)\{–4,4,7,8\}\newline(B){–11,–1,3,10}(B)\{–11,–1,3,10\}\newline(C){3,4,7,8}(C)\{3,4,7,8\}\newline(D){–7,–4,4,8}(D)\{–7,–4,4,8\}
Get tutor helpright-arrow

Posted 4 months ago

Question
What is the domain of this function?\newline(10, –3)(2, –9)(–5, 8)(3, 1)(10,\,–3)(2,\,–9)(–5,\,8)(3,\,1)\newlineChoices:\newline[{–5,2,3,10}][{–9, –3,1,8}][{1,2,3,10}][{–10, –5,2,3}][\{–5,2,3,10\}][\{–9,\,–3,1,8\}][\{1,2,3,10\}][\{–10,\,–5,2,3\}]
Get tutor helpright-arrow

Posted 2 months ago

Question
What is the domain of this function?\newline(1,–4)(9,–12)(–6,11)(7,5)(1,–4)(9,–12)(–6,11)(7,5)\newlineChoices:(A){–6,1,7,9}\newline\\(A)\{–6,1,7,9\}\newline(B){–12,–4,5,11}(B)\{–12,–4,5,11\}\newline(C{5,1,7,9}(C\{5,1,7,9\}\newline(D){–9,–6,1,7}(D)\{–9,–6,1,7\}
Get tutor helpright-arrow

Posted 2 months ago

View all (74)